EARTH
DYNAMICS
|
Contents |
Earth Dynamics
I have been continually amazed that the simplicity with which Earth expansion answers so much of the Earth's evolution has been so delayed in universal adoption (Vogel, 1983).
When Hilgenberg (1933) fitted the continental crust onto a small Earth globe he operated within the outlines of the continents and continental shelves known at the time. Vogel (1990) considered that, athough fairly exact in several regions, the totality of Hilgenberg's result was not entirely convincing because, especially in the Indian, Pacific and Arctic Ocean regions, numerous gaps and overlaps appeared between continental fragments. Suppositions for the small Earth reconstructions of Hilgenberg, as well as Barnett (1962), Brösske (1962), Creer (1964), Dearnley (1965a), Owen (1976) and Shields (1979) in particular, have also changed fundamentally with the discovery and investigation of the world-wide system of mid-oceanic ridges, in connection with sea-floor spreading, allowing a far better quantitative approach to be made towards small Earth reconstructions.
For the majority of small Earth reconstructions made since then, it has been customary to adopt the 200 metre isobath as representing the continental margin (eg. Hilgenberg, 1933; Barnett, 1962; Vogel, 1983). As previously mentioned, this results in a small Earth globe approximately 55% of the present Earth radius. The 200 metre isobath was gauged by Worsley et al (1984) as the relative position of the worlds ice-free shelf break, and assumes a constant or uniformly accreting continental crust, and constant ocean water volume. In contrast Owen (1976) adopted the 1000 metre isobath for his modeling, which gave a small Earth globe approximately 80% of the present radius.
Vogel (1983), from his calculations, realized however that it was theoretically possible for the continents, without shelves, to fit together as an even smaller Earth globe, approximately 40% of the size of the present Earth (Figure 7). He considered that the continental shelves could have formed only after the brittle upper crust had broken into pieces (Kremp, 1992).
Mörner (1981) in an analysis of Cretaceous sea level data demonstrated that, besides local crustal movements and global tectono-eustatic changes, there were also considerable geoidal tectono-eustatic changes during the Cretaceous period. These were considered to represent large-scale latitudinal north-to-south migrating geoidal-eustatic highs and lows, which Mörner attributed to be connected with the varying mass distribution due to continental drift [plate tectonics]. This change in mass distribution could however be attributed equally to a changing surface curvature during expansion and crustal equilibration of the Earth. This suggests to me that caution needs to be applied in the adoption of a particular isobathic contour as representative of past continental margins.
Lithospheric budget
In all modern global tectonic concepts, generation of new lithosphere during the Mesozoic and Cenozoic Eras is generally considered to have been continuously created along mid-oceanic sea floor spreading centres; within back-arc basins; and to a limited extent as continental magmatic intrusions; oceanic magmatic intrusions along transform fault systems or fracture zones; volcanic accretion along island arcs; and, less noticeably, as crustal extension within intracontinental sedimentary basins (eg. Morgan, 1968; Hawkins et al, 1984; Irvine, 1989; Lundberg & Reed, 1991; Phipps Morgan, 1991; Cloos, 1993). Whereas, during the Palaeozoic to Precambrian eras indications are that continental crustal thinning and extension may have been the dominant process (eg. Glikson, 1979, 1980; Neiman, 1983, 1990; Bozhko, 1987; Vogel, 1990).
With the completion of oceanic magnetic isochron coverage during the mid 1980s to the Present (eg. Larson et al, 1985; CGMW & UNESCO, 1990) a method of quantification of the Earth's lithospheric budget is now available to constrain both plate configuration and distribution of surface area with time.
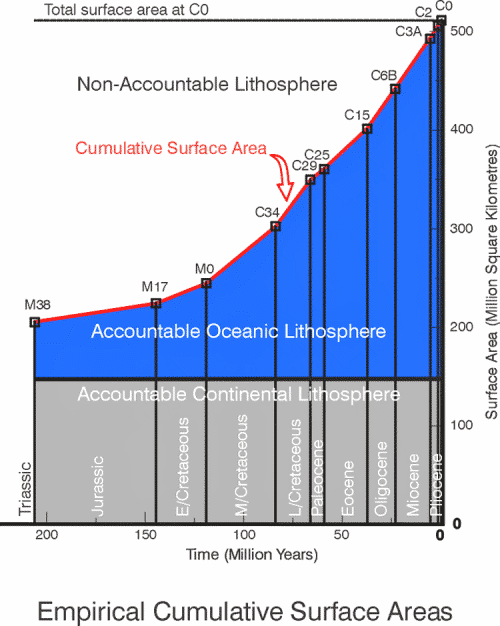
Within the context of this paper determination of the Earth's present day lithospheric budget was originally based on the oceanic magnetic isochron mapping of Larson et al (1985), and subsequently verified using the mapping of CGMW & UNESCO (1990). In order to remove projection distortions inherent in these maps the isochron information was first manually transferred onto true-to-scale "24-gore" sinusoidal equal-area map projection, and continental outlines similarly transferred from a "Replogue, World Ocean Series" globe, with a natural scale of 1:41,849,600. The lithospheric budget was determined by computing the cumulative surface areas of oceanic magnetic isochron data, to the limit of the data shown (chron M38: approximately 205 million years).
The "24-gore" sinusoidal base map derived from the Larson et al
(1985) isochron map was digitised using a Computer Aided Design (CAD) Graphical
Design System (GDS) software package, and surface areas of successive chron
intervals calculated. The raw data is summarised in Table 1, and
a graphical plot of empirical cumulative surface area against time is shown
in
Figure 15.
|
|
|
|
|||
| Chron | Myr (x106) | dS (x107 km2) | S dS (x107 km2) | S=S0-SdS (x107 km2) | R (km) |
| 0 | 0 | 0 | 0 | 51.0000 | 6370.80 |
| C2 | 0-1.9 | 0.5342 | 0.5342 | 50.4658 | 6337.15 |
| C3a | 1.9-5.9 | 1.3328 | 1.8670 | 49.3300 | 6265.43 |
| C6b | 5.9-23.0 | 4.9213 | 6.7883 | 44.2117 | 5931.49 |
| C15 | 23.0-37.7 | 4.1624 | 10.9507 | 40.0493 | 5645.37 |
| C25 | 37.7-59.2 | 4.1649 | 15.1156 | 35.8844 | 5343.77 |
| C29 | 59.2-66.2 | 1.0462 | 16.1618 | 34.8382 | 5265.30 |
| C34 | 66.2-84.0 | 4.7956 | 20.9574 | 30.0426 | 4889.49 |
| M0 | 84.0-118.7 | 5.6758 | 26.6332 | 24.3668 | 4403.46 |
| M17 | 118.7-143.8 | 1.9348 | 28.5680 | 22.4320 | 4225.02 |
| M38 | 143.8-205 | 1.9386 | 30.5066 | 20.4934 | 4038.31 |
Table 1 Empirical surface area data derived from Larson et al (1985). Areas digitised and calculated using a CAD based Graphical Design System software package. Arbitrarily assigned errors are ±5%. Where: S = total surface area at end of chron interval, S0 = present surface area = 5.1x108 km2 (Stacey, 1977), dS = surface area of chron interval, S dS = cumulative surface area, R = palaeoradius.
The accuracy of computed values presented in Table 1 depend primarily on the accuracy of the original mapping, accuracy maintained during cartographic transferral of original isochron data onto the "24-gore" sinusoidal base maps, and limitations of the graphical software package used for calculation of areas. The limitations of the Larson et al (1985) primary base map are unknown, and were accepted without modification beyond addition of isochron data in the Arctic Ocean region using CGMW & UNESCO (1990), not previously covered by Larson et al (1985).
To minimise subsequent errors, computations of digitised areas were checked by comparing the total area of the "24-gore" sinusoidal base map against the actual Earth surface area (Stacey, 1977), and isochron surface area values adjusted on a pro-rata basis. The variation between computed and actual values was found to be less than 5%. An arbitrary relative error of 5% was therefore chosen, as a measure of the goodness of fit.
Figure 15 depicts the Earth's lithospheric budget for the past 205 million years, showing accountable oceanic and continental lithosphere located below the cumulative surface area curve, and non-accountable, or "presumed pre-existing" lithosphere located above the cumulative surface area curve. A horizontal line is also shown at the top of the figure depicting the projected total Earth surface area at the Present chron C0.
To account for the cumulative distribution of the Earth's oceanic and continental crust for the past 205 million years, the lithospheric budget shown in Figure 15 can be explained by consideration of the three main global tectonic theses, namely, the data and graphical display represents:
Figure 16 a constant lithospheric budget whereby lithosphere generated at spreading centres is continuously disposed of along subduction zones, i.e. plate tectonics;
Figure 17 a partially cumulative lithospheric budget where excess lithosphere is disposed of along subduction zones, ie. the compromise theses of Owen (1976) and Steiner (1977) or;
Figure 18 a cumulative lithospheric budget whereby lithosphere generated at spreading centres is cumulative with time, and the accountability is compensated for by an increase in palaeoradius, i.e. Global Expansion Tectonics .
It is now almost universally appreciated that oceanic lithosphere generated at spreading centres, on a constant radius Earth, must be disposed of in order to maintain a balanced lithospheric budget. For an expanding Earth however, the surface area is considered to have been cumulative, and therefore the lithospheric budget is also considered cumulative with time.
For the purpose of the remainder of this paper, in order to investigate the oceanic data on an expanding Earth, it is assumed that the Earth's post-Jurassic lithospheric budget has been cumulative with time. Similarly the surface area of oceanic lithosphere, as represented by the oceanic magnetic isochron data, is assumed to be fully fixed in the rock record
Extrapolation to the Pre-Jurassic
In the concept of Global Expansion Tectonics, prior to the Early Jurassic modern deep ocean basins did not exist and, all continental lithosphere was united to form a single Pangaean supercontinent enclosing the Earth at a much reduced palaeoradius. Oceanic areas were represented by shallow intra or epi-continental seas, with deposition of sediments within "geosynclinal" sedimentary basins masking all evidence of sea floor spreading. The volume of hydrosphere, and atmosphere, is considered to have increased with time, in sympathy with volume of oceanic lithosphere. Constraint of palaeoradius using oceanic surface areas during the Pre-Jurassic is not possible, and reliance must be placed on empirical reconstruction of continental lithosphere to constrain palaeoradius .
The very important question then arises. Do we consider that the pre-Jurassic continental lithospheric budget remained static or, do we consider that an increase in continental lithospheric surface area with time is a natural consequence of core-mantle expansion, prior to continental breakup, dispersal, and formation of modern deep oceanic basins? [The full quantification of this statement is currently being researched, and will be extensively elaborated on in due course, sufficient to say at this stage that investigations to date are extremely encouraging.]
If, like the post-Jurassic oceanic lithosphere, we adopt the later suggestion, then preliminary empirical small Earth models for the Palaeozoic and Precambrian indicate that the primordial Earth size for the Archaean was approximately 1700 kilometres radius.
Figure 19 Palaeoradius of the Earth from the Archaean to the Present derived from published oceanic isochron data and continental geology.
As shown in Figure 19 the palaeoradius during the Precambrian remained relatively static throughout the Archaean to late Mesoproterozoic, increasing by approximately 60 kilometres radius during this time. This accords well with published literature (e.g. Glikson, 1980) which suggests that there is no evidence for Earth expansion prior to the Mesoproterozoic, and the scarcity of evidence for horizontal crustal movements places constraints on the extent of expansion prior to the upper Proterozoic. The dominance of tensional tectonics during the Archaean and Proterozoic however suggested to Glikson (1980) that, some degree of expansion may have occurred by crustal dilation associated with faulting and rifting.
Glikson (1980) considered that the evolution of Proterozoic mobile belts (eg. Davies & Windley, 1976; Katz, 1976) within a hot and ductile sialic crust may have involved significant degrees of crustal expansion. Secular geochemical trends for the Precambrian suggested also that, evolution of large oceanic domains may have only commenced at about 600 million years, whereas those domains aged between 1 billion and 600 million years constituted "narrow gaps". The significance of major Precambrian thermal peaks (eg. Dearnley, 1966, 1969) and geochemical trends, led Glikson (1980) to conceive the following correlation between those peaks and crustal tectonic events in the Precambrian:
2.7-2.5 billion year peak: completion of Archaean sima-sial transformation process;
1.9-1.7 billion year peak: peak of tensional tectonic events within intrasialic mobile belts, possibly signifying a crustal expansion episode and;
1.0 billion year peak: a major crustal tension phase, involving intrasialic rifting and opening of oceanic gaps, signifying onset of large-scale horizontal global tectonics.
The Global Expansion Tectonic Precambrian Earth expansion process is therefore considered to have been very much subdued during Archaean crustal formation, Proterozoic crustal fragmentation, and Proterozoic to Palaeozoic basin development, prior to development of intra or epi-continental Palaeozoic "geosynclinal’ sedimentary basins and post- Mesozoic crustal breakup and continental dispersal. From the expanding Earth cumulative surface area data (Table 1), in conjunction with preliminary continental data, it is therefore possible to establish a mathematical relationship defining the rate of change in palaeoradius, in order to accurately constrain the Earth expansion process with time.
Determination of palaeoradius
Determination and quantification of the palaeoradius of the Earth has been one of the basic requirements of any expanding Earth thesis. Prior to completion of the geological and geochronological coverage of all oceanic basins during the 1980s, palaeoradius was determined by empirical modeling (eg Hilgenberg, 1933; Barnett, 1962; Brösske, 1962; Creer, 1964, 1965, 1967; Dearnley, 1965; Owen, 1976; Shields, 1979, 1983; Schmidt & Embleton, 1981; Vogel, 1983), palaeomagnetism (eg. Egyed, 1960; Carey, 1961; Cox & Doell, 1961a, 1961b; Ward, 1963; van Hilten, 1963, 1968; McElhinny & Brock, 1975), measurement of areas of sea floor spreading (eg. Berger & Winterer, 1974; Steiner, 1977; Sclater et al, 1981; Blinov, 1983), mantle plume separation (eg. Stewart, 1976) and astronomical observation (eg. Gorai, 1983; Talobre, 1983; Zhi-Geng, 1990), however in all cases it was not possible to accurately constrain palaeoradius with time.
Consideration of available literature suggests that there has been no unified opinion amongst authors for the rate at which the Earth may be expanding (eg. Carey, 1975, 1976, 1988; Owen, 1976; Steiner, 1977; Blinov, 1983; Vogel, 1983, 1990; Zhi-Geng, 1990), contracting (eg. Meyerhoff et al, 1992), pulsating (eg. Milanovsky, 1980), rhythmical (eg. Smirnoff, 1992) or static (eg. McElhinny et al, 1978; Parsons, 1981, 1982; Stewart, 1983) with time. A comprehensive review of determinations of rate of Earth expansion is given in Wesson (1973).
Depending on which method was used to determine palaeoradius, (eg. empirical modeling, palaeomagnetism, mantle plume separation, astronomical, direct measurement of areas, or satellite laser ranging), reference is made of a "large" expansion of the Earth (eg. Hilgenberg, 1933; Carey, 1958; Heezen, 1959; Neiman, 1962; van Hilten, 1963; Blinov, 1983; Vogel, 1984) equated with a rate of expansion of between 0.4 to 2 cm per year. There are also descriptions of "gross" radial expansion (eg. Yarkovskii, 1899; Romanov, 1966) reaching several metres per year, and another widely held opinion which allows for "small" to "negligible" (eg. Cox & Doell, 1961a, 1961b; Ward, 1963; Egyed, 1969; Dooley, 1973; McElhinny et al, 1978, Weijermars, 1986) radial expansion of up to 1 mm per year.
Figure 20 shows a comparison of published rates of expansion for the main methods and clearly shows the large variation in results, from slow linear rates advocated by palaeomagnetism to fast exponential rates indicated by empirical modeling of oceanic lithosphere. Empirical modeling of continental lithosphere and mantle is also shown for comparison. It should be noted that this is the first time that continental lithosphere has been used in determining palaeoradius prior to the Palaeozoic. It is acknowledged that these preliminary results are speculative at this stage, and depend on completion of current continental research.
The mathematical relationship for the rate of change of palaeoradius from the Archaean to the Present was established by including Post-Jurassic oceanic data (Table 1) and Pre-Jurassic empirical modeling (Figure 19). By considering the exponential increase in palaeoradius derived from oceanic and continental lithosphere, the method of least mean squares was used to calculate the gradients of curves of best fit. The mathematical equation integrates to:
Ra = (R0 - Rp)ekt + Rp (Equation 1)
where: Ra = ancient palaeoradius of the Earth, R0 = present radius of the Earth, Rp = primordial Earth radius = approx. 1700 km, e = exponential, t = time before the present (negative), k = a constant = 4.5366 x 10-9/yr.
This equation represents a mathematical relationship defining the curve of best fit for an exponential increase in the Earth's palaeoradius from the Archaean to the present, derived from both post-Jurassic empirical cumulative surface area data, and pre-Jurassic empirical modeling of Palaeozoic and Proterozoic continental lithosphere. Comparison of the calculated exponential increase in the Earth's palaeoradius with the empirical palaeoradius data (Figure 19) suggests that the mathematical equation satisfactorily reflects changes in the Earth's radius with time, and indicates that Earth expansion has been most active from the Mesoproterozoic to the present.
Cause of Earth expansion
Concerning the physical cause of Earth expansion Creer (1965) maintained that, "we should beware of rejecting the hypothesis of Earth expansion out of hand on grounds that no known sources of energy are adequate to explain the expansion process". Creer further considered that, "it may be fundamentally wrong to attempt to extrapolate the laws of physics as we know them today to times of the order of the age of the Earth and of the Universe".
Carey (1976) stated that, he "may not necessarily be expected to know" the cause of Earth expansion since the answer could only be expected to be known if all relevant fundamental physics were already known. This remains equally true today, as in the past, since historically, in the evolution of knowledge, empirical phenomena have commonly been recognised long before their cause or reason has been understood.
Egyed (1963) summarized hypotheses proffered on the cause of Earth expansion since the theory of Earth expansion first gained recognition in the 1890s, and Wesson (1973) gave a comprehensive review of the cosmological implications of an expanding Earth. Carey (1983a) provided a comprehensive list of authors who have contributed to the causes of Earth expansion and recognised five main reoccurring themes:
a pulsating Earth (eg. Khain, 1974; Steiner, 1967, 1977; Milanovsky, 1980; Smirnoff, 1992; Wezel, 1992), where cyclic expansion of the Earth opened the oceans and contractions caused orogenesis. The proposal fails to satisfy exponentially waxing expansion and Carey (1983a) considered the theme to have arisen from the "false axiom" that orogenesis implies crustal contraction, and saw no compelling evidence for intermittent contractions;
meteoric and asteroidal accretion (eg. Shields, 1983a, 1988; Dachille, 1977, 1983; Glikson, 1993). Rejected by Carey (1983a) as the primary cause of Earth expansion since expansion should then decrease exponentially with time, nor was ocean floor spreading explained;
constant Earth mass, with phase changes of an originally super-dense core (eg. Lindemann, 1927; Egyed, 1956; Holmes, 1965; Kremp, 1983). Rejected by Carey (1983a) as the main cause of Earth expansion [however reviewed favourably within this page] because he considered the theme to imply too large a surface gravity throughout the Precambrian up untill late in the Palaeozoic, which he further considered was denied by several kinds of evidence (eg. Stewart, 1977, 1978, 1983).
secular reduction of the universal gravitation constant G (eg. Ivanenko & Sagitov, 1961; Dicke, 1962; Jordan, 1969; Crossley & Stevens, 1976; Hora, 1983). Such a decline of G would cause expansion through release of elastic compression energy throughout the Earth and phase changes to lower densities in all shells (Carey, 1983a). Carey again however rejected the proposal as the main cause of expansion for three reasons; that formerly surface gravity would have been unacceptably high; that the magnitude of expansion is probably too small; and the arguments for such reduction in G were considered not to indicate an exponential rate of increase and;
a cosmological cause involving a secular increase in the mass of the Earth as proposed by Hilgenberg (1933), Kirillov (1958), Blinov (1973, 1983), Wesson (1973), Carey (1976), Neiman (1984, 1990) and Ivankin (1990).
Carey (1983a) considered that each of the proposals briefly indicated above are soundly based and may have contributed to Earth expansion, however he considered that, because of the limitations of surface gravity in the past, there may be no alternative to an exponential increase of Earth mass with time. Where the excess mass came from was considered at length (Carey, 1983a; 1988; 1994) and he suggested that Einstein's equation E=Mc2 implies that matter and energy are interconvertable, and therefore matter is the antithesis of energy. The instant that new matter, of mass M, appears in the Universe, the potential energy E of the whole Universe increases by Mc2. Carey (1983a) considered that new mass added to the Earth must appear deep within the core, and the ultimate cause of Earth expansion, Carey concluded, must be sought in a cosmological expansion of the Universe.
More recently Kremp (1992) suggested that new geophysical evidence indicates that the Earth has been growing rapidly in the last 200 million years. Kremp indicated that seismologists have located the existence of a zone, about 200 to 300 kilometres thick at the base of the mantle, designated some 40 years ago as the "D" region. Yuen & Peltier (1980) as well as Boss & Sacks (1985) postulated the existence of a substantial flow of heat across the core-mantle boundary and concluded that if whole-mantle convection occurs in the Earth's mantle then this "D" region should be the lowest thermal boundary layer of the whole-mantle convection circulation system. With the temperature of the outer core of the core-mantle boundary estimated to be about 800 degrees higher (Lay 1989) than the "D" layer of the mantle, or perhaps even 1500 degrees higher (Williams et al 1987), Kremp (1992) concluded that this thermal increase in the outer core may be a fairly recent process forcing a rapid expansion of the Earth during the Hercynian and Alpine orogenies.
Once again it is considered that caution should be excercised in consideration of the cause of Earth expansion for fear of scientific ridicule. Old data and old modeling techniques suggested that there were limitations to surface gravity in the past (Stewart, 1977, 1978) and hence there may be no alternative to an exponential increase of Earth mass with time. It is now time to apply modern global tectonic principles to quantify the cause of Earth expansion.
Kinematics of Earth expansion
Previous investigations into the kinematics of Earth expansion with time have been limited by a lack of any quantitative mathematical basis for determination of palaeoradius. Investigations tended to dwell on the theoretical aspects of a potential Earth expansion, such as: determination of energy changes in an expanding Earth (Beck, 1960, 1969; Hora, 1983); variation of the gravitational constant G (Wilson, 1960; Birch, 1968; Jordan, 1969; Wesson, 1973; Crossley & Stevens, 1976; McElhinny et al, 1978); limits to palaeogravity (Stewart, 1977, 1978, 1983; Blinov, 1983); the Earth's tectonic development (Dearnley, 1965a, 1965b, 1969; Milanovsky, 1978, 1980; Kuznetsov, 1983, 1984); thermal expansion (Pfeufer, 1983; Tassos, 1983); lower mantle convection (Walzer & Maaz, 1983); astronomical observations (Tryon, 1983; Zhi-Geng, 1990); variations of the synodic month from palaeontological evidence (Holmes, 1965; Pannella et al, 1968; van Diggelin, 1976; Stewart, 1983); and palaeogeographical distribution of land and sea (Egyed, 1956; Holmes, 1965; Termier & Termier, 1969; Hallam, 1971; Davidson, 1983). Carey (1975) provides a comprehensive review of most of these topics.
An investigation into the kinematics of an Earth undergoing exponential expansion with time will be undertaken using the empirically derived Equation 1 for palaeoradius, derived from empirical oceanic and continental data shown in Table 1 and Figure 19. Equation 1 is accepted as satisfactorily reflecting changes in the Earth's palaeoradius from the Archaean to the Present. The primary assumptions being that, oceanic lithosphere generated during sea floor spreading, and continental lithosphere, is fully fixed in the rock record, and continues to increase the surface area of the Earth with little or no requirement for removal of lithosphere by subduction.
Known parameters for an Earth at time t0 (Present) (Stacey,
1977), and calculated parameters for an Earth at time t4000
(Early Archaean), established from Equation 1, are:
|
|
|
| Palaeoradius R0 = 6.3708 x 106 m | R4000 = 1.7 x 106 m |
| Surface Area S0= 5.1000 x 1014 m2 | S4000 = 3.6317 x 1013 m2 |
| Volume V0 = 1.0830 x 1021 m3 | V4000 = 2.058 x 1019 m3 |
| MassM0 = 5.9730 x 1024 kg | |
| Mean Density D0 = 5.5150 x 103 kg/m3 | |
| Universal Gravity G0 = 6.6732 x10-11 m3 kg-1 sec-2 | |
| Surface Gravity g0 = 9.780317 m sec-2 |
Radius, circumference, surface area and volume
Secular variation curves for the time interval t+300 to t-4000
are shown in Figure 21 for the variables radius, circumference,
surface area and volume, calculated using the following equations:
| Ancient Radius Ra | = R0 + dR = (R0 - Rp)ekt + Rp |
| Ancient Circumference Ca | = C0 + dC = 2p ((R0 - Rp)ekt + Rp) |
| Ancient Surface Area Sa | = S0 + dS = 4p ((R0 - Rp)ekt + Rp)2 |
| Ancient Volume Va | = V0 + dV = 4p /3 ((R0 - Rp)ekt + Rp)3 |
Figure 21 Palaeoradius, circumference, surface area and volume secular variation curves for the Earth undergoing exponential expansion from the Archaean to the Present.
The curves shown in Figure 21 demonstrate, assuming lithosphere is fully fixed in the rock record with no requirement for subduction, the accelerating increase in all four variables since breakup of the Pangaean supercontinent during Early Jurassic. The curves represent the secular variation of each variable, under conditions of an exponential expansion of the Earth with time, and effectively quantify the empirical results of Hilgenberg (1933), and Vogel (1983, 1990), derived from small Earth modeling.
Mass, Density and Surface Gravity
The kinematics of mass, density and surface gravity, assuming a simplistic
spherical Earth model with a homogeneous mass and density distribution,
are governed by the standard equations:
| Density D | = Mass/Volume |
| Surface gravity g | = GM/R2 |
Where: G = universal gravitation, M = mass, R = palaeoradius
Because the density equation contains two unknown variables; mass M and density D, three possible scenarios exist for an expression representing the kinematics of mass, density and surface gravity with time (Figure 22):
mass curve A remains constant, requiring both density curve B and surface gravity curve C to decrease exponentially with time;
density curve E remains constant, requiring both mass curve D and surface gravity curve F to increase exponentially with time or;
mass, density and surface gravity are variable with time, and plot within the bounds of curves A, B, C, D, E and F.
The effects of each of these scenarios is demonstrated in Figure 22. Density at constant Earth mass decreases exponentially from a peak of approximately 290 grams/cm3 during the Archaean and Palaeoproterozoic, to a Present value of 5.52 grams/cm3, and approachs zero at about 300 million years in the future. Mass at constant density increases exponentially from approximately 1.1 x 1023 kilograms during the Archaean and Mesoproterozoic, to a Present value of 5.97 x 1024 kilograms, and 182 x 1024 kilograms at about 300 million years in the future.
Assuming the universal gravitation G to be constant the effects of surface gravity under the extreme conditions of constant mass and constant density with time is also shown in Figure 22. For a constant mass surface gravity decreases exponentially from a peak of approximately 138 metre sec-2 during the Archaean and Palaeoproterozoic, 95 metre sec-2 during the Cambrian, to a Present value of 9.8 metre sec-2, and approaches 1 metre sec-2 at about 300 million years in the future. For a constant density and exponentially increasing mass with time, surface gravity increases exponentially from approximately 2.6 metre sec-2 during the Archaean and Mesoproterozoic, to approximately 31 metre sec-2 at about 300 million years in the future.
Figure 22 Secular variation curves for density, surface gravity and mass demonstrating the kinematics of the Earth under conditions of (a) constant mass and, (b) constant density.
At this stage it is considered that the exact kinematics of both mass and density, and hence surface gravity, with time is indeterminate without additional cosmological constraints. As previousley mentioned Carey (1983a) considered that, because of the limitations of surface gravity in the past, there may be no alternative to an exponential increase of Earth mass with time, however the reality displayed in Figure 22 suggests otherwise. At a constant mass the Earth's density during the Archaean approximates densities for the Present Sun, and the decline in both density and surface gravity for the future accords with densities and surface gravity of the Present outer planets. An exponential increase in mass, even subdued by a variation in density, appears to me to be contrary to the kinematics of the rest of the present solar system. It may be that, in reality, we have no alternative than to concede that mass may be constant with time.
Secular rate of Earth expansion
The secular rate of Earth expansion may be defined as an incremental increase in the physical dimensions of the Earth with time. These can be quantified by consideration of the variables radius, circumference, surface area and volume. Mass, density and surface gravity are considered speculative at this stage however tentative values will be given for reference only.
The application of Equation 1, as an expression of the empirically
derived curve of best fit for an exponential increase in palaeoradius,
enables the secular rate of Earth expansion to be determined for the following
variables:
| dR/dt | = R1/t1 - R2/t2 = ((R0 - Rp)ekt1 + Rp)/t1 - ((R0 - Rp)ekt2 + Rp)/t2 |
| dC/dt | = C1/t1 - C2/t2 = (2p ((R0 - Rp)ekt1 + Rp))/t1 - (2p ((R0 - Rp)ekt2 + Rp))/t2 |
| dS/dt | = S1/t1 - S2/t2 = (4p ((R0 - Rp)ekt1 + Rp)2)/t1 - (4p ((R0 - Rp)ekt2 + Rp)2)/t2 |
| dV/dt | = V1/t1 - V2/t2 = (4p /3 ((R0 - Rp)ekt1 + Rp)3)/t1 - (4p /3((R0 - Rp)ekt2 + Rp)3)/t2 |
And, assuming a constant Earth mass with variable density
and surface gravity;
|
dM/dt
|
= 0 |
| dD/dt | = D1/t1 - D2/t2 = (M0/(4p /3 ((R0 - Rp)ekt1 + Rp)3))/t1 - (M0/(4p /3 ((R0 - Rp)ekt2 + Rp)3))/t2 |
| dg/dt | = g1/t1 - g2/t2 = GM/((R0- Rp)ekt1 + Rp)2/t1- GM/((R0 - Rp)ekt2 + Rp)2/t2 |
Where: R = radius, C = circumference, S = surface area, V = volume, M = mass, D = density, g = surface gravity, k = 4.7677 x 10-9/yr [4.6579 x 10-9/yr], t = time before the present (negative)
Using the Equations above for the interval of time t0(Present)
to t1 (minus one year) the present rate of Earth expansion
calculates as:
| dR1/dt1 | = 2.10 cm/year |
| dC1/dt1 | = 13.0 cm/year |
| dS1/dt1 | = 3.50 km2/year |
| dV1/dt1 | = 10,000 km3/year |
| dM1/dt1 | = 0 kg/year |
| dD1/dt1 | = - 1 x 10-4 kgm-3/year |
| dg1/dt1 | = - 6.50 x 10-8 msec-2/year |
The secular rates of Earth expansion calculated above compare favourably with Steiner (1977) who estimated a global rate of areal sea floor spreading, during the past 5 Ma, of 3.19 km2/yr, with a rate of radial expansion calculated from this of 1.99 cm/yr. Garfunkel (1975) similarly calculated an areal spreading rate of 3.15 km2/yr, and Parsons (1982) 3.45 km2/yr. These figures are further substantiated by calculations of intercontinental chord lengths from VLBI and SLR satellite measurements of (Christodoulidis et al, 1985; Cohen et al, 1985) with the rate of increase in Earth radius calculated by Carey (1986) to be 2.4 ±0.8 cm/yr, and recently 1.8 cm/yr from VLBI and SLR geodetic measurements (Robaudo & Harrison, 1993).
The rate of equatorial and meridional circumferential expansion of the Earth was calculated by Blinov (1983) by considering the relative plate motions in the Pacific, Atlantic and Southern Oceans, giving a value of 12 cm/yr, and a rate of radial expansion of 1.91 cm/yr. Using magnetic isochron data, Steiner (1977) calculated the average "ocean-basin-wide" sea floor spreading rates for the past 5 Ma to be approximately 9.6 cm/yr for the Pacific Ocean, 7.6 cm/yr for the Indian Ocean, and 4.3 cm/yr for the Atlantic Ocean. These, although not equatorially or meridionally aligned, are in close agreement with the figure for increase in equatorial circumference calculated above.
Discussion
In order to accurately quantify any variation in the Earth's palaeoradius, and constrain plate configuration with time, it was argued that it is necessary to take into account the area and pattern of oceanic lithospheric and continental crustal generation, as portrayed in maps such as Larson et al (1985) and CGMW & UNESCO (1990). What the oceanic magnetic isochron maps are showing is the spatial and temporal distribution of oceanic lithosphere within each of the oceanic basins. By moving backwards in time successively younger chron intervals from across active spreading ridges, such as the Mid-Atlantic and Indian Ocean ridges, must be removed and each of the remaining chron intervals reunited along their common spreading ridges.
The conclusions drawn from consideration of the Earth's lithospheric budget, measured from maps such as Larson et al (1985) and CGMW & UNESCO (1990) however, hinge on three mutually exclusive considerations, namely:
the surface area of oceanic lithosphere measured represents the total area of new oceanic crust generated and preserved during the interval of time under study, hence there has been an expansion of the Earth with time in keeping with Global Expansion Tectonics;
the surface area of oceanic lithosphere measured represents the remnant oceanic crust remaining after subduction has occurred up to the present day, hence there has been no expansion of the Earth with time in keeping with plate tectonics or;
the surface area of oceanic lithosphere measured represents the remnant oceanic crust remaining after partial subduction on an expanding Earth in keeping with the conclusions of Owen (1976) and Steiner (1977).
Consideration (1) forms the basis of Global Expansion Tectonics
and the mathematical equation derived from the oceanic crustal data in
particular represent a confirmation and refinement of earlier modeling
studies by Hilgenberg (1933), Barnett (1962, 1969) and Vogel (1983, 1984,
1990). The adoption of this assumption to the oceanic crustal mapping of
Larson et al (1985) and CGMW & UNESCO (1990), in conjunction
with empirical continental geological data, has enabled the variation in
palaeoradius of the Earth to be mathematically quantified as:
|
PalaeoradiusRa |
= (R0 - Rp)ekt + Rp (Equation 1) |
Where: Ra = ancient palaeoradius of the Earth, R0 = present radius of the Earth, Rp = primordial Earth radius = approx. 1700 km [possibly 1800 km], e = exponential, t = time before the Present (negative), k = 4.5366 x 10-9/yr
Equation 1, assuming the assumptions used to derive this equation are correct, is considered to be the "fundamental equation" for Global Expansion Tectonics, enabling the kinematics of an Earth undergoing exponential expansion, from the Archaean to the Present, to be readily determined. The kinematics of Earth expansion suggests that the controlling influence on Earth expansion may not have been a secular increase in mass with time, as suggested by Carey, (1983a) and others, but instead, a secular decrease in density and surface gravity under conditions of constant mass accords better with the kinematics of the rest of the solar system. The ultimate cause of Earth expansion must however be considered intimately related to a cosmological expansion of the Universe.
In contrast, consideration (2) is fundamental to plate tectonics and cannot be reconciled with Global Expansion Tectonics. Direct measurements across the Pacific Ocean, using satellite laser ranging to detect the relative plate motions (Christodoulidis et al, 1985; Cohen et al, 1985), indicated to Owen (1992) and Carey (1986, 1994) that the preliminary data, in particular the chord length increase between Australia and South America, are not accountable for an Earth of constant dimensions. A value of 2.4 ± 0.8 cm/yr for rate of increase in Earth radius (Carey, 1986), calculated from satellite laser ranging measurements, and 1.8 cm/yr from VLBI and SLR geodetic measurements (Robaudo & Harrison, 1993), while speculative, compare favourably with the 2.1 cm/yr estimated above, using the empirical sea floor surface area data of Larson et al (1985) and CGMW & UNESCO (1990). These values are also comparable with 1.99 cm/yr calculated from Steiner (1977) and 1.91 cm/yr from Blinov (1983). This secular increase in Earth radius is considered adequate to account for all of the ocean floor growth since the Early Cretaceous, and continental growth since the Archaean, without the need for consideration of subduction of oceanic lithosphere.
Similarly consideration (3), which accommodates partial subduction on an expanding Earth, in keeping with the conclusions of Owen (1976) and Steiner (1977), is also rejected. Even though Owen (1976) went to great lengths to ensure cartographic accuracy during modeling, it is considered that the 1000 metre isobath adopted by Owen as the edge of the continental outlines is too inflexible, and in many cases non-representative of the wide variation of continental margins present throughout the world. Geoidal tectono-eustatic changes during the Cretaceous period (Mörner 1981) indicate a need for caution in adoption of a particular isobathic contour, while empirical modeling by Hilgenberg (1933), Brösske (1962), Barnett (1962, 1969), Creer (1965) and Vogel (1983, 1984, 1990) demonstrate that small Earth models are feasible at much smaller radii than indicated by both Owen (1976) and Steiner (1977). These small Earth models empirically negate the need for partial removal of excess lithosphere by subduction processes.
The adoption of consideration (1) above, as representing the accumulation of oceanic and continental lithosphere on an Earth undergoing exponential expansion since the Archaean, is considered justifiable. Consideration will now be given to testing Global Expansion Tectonics by way of quantitative small Earth modeling.