Geological Implications of an Expanding Earth

the fit of the continents on a smaller Earth appeared to be too good to be due to coincidence and requires explaining (Creer 1965)

Acceptance of the theory of Earth expansion was envisaged by researchers, such as McElhinny et al (1978) and Schmidt & Clark (1980), to be thwarted by major obstacles which "outnumber the evidence in favour". Perceived problems included an explanation for the existence of the two very different extensional structures exposed on the oceanic floors: the mid-oceanic ridges and; the trench-arc/back-arc zones characterised by very different seismicity and volcanism, the problem of atmospheric and hydrospheric accumulation on an expanding Earth, and the adaptation of palaeomagnetics to a constantly variable Earth radius.

Scalera (1990) asked, why should a body which is expanding develop huge diapiric extensional basins with a very deep source, as testified by the earthquake foci pattern, while elsewhere, very shallow extensional ridges with an associated shallow seismicity implies a great complexity of the global expansion process? Similarly Brunnschweiler (1983), in a paper dealing with the evolution of geotectonic concepts in the past century, considered that Earth expansion was essentially a radial movement and therefore its tangential plate displacements are only apparent, not real. The possibility of orogenesis developing under these conditions of radial expansion was discounted by Rickard (1969) because the necessary vertical movements did not appear to explain the observed compressional features.

Weijermars (1986) considered that, for a pre-Jurassic small Earth with a continuous continental crust, a large expansion process implies that the entire Earth would have been covered by an ocean with an average depth of 6.3 kilometres. This implication disagrees with the maximum possible sea-level rise of only a few hundred metres above the present, inferred from the stratigraphic record (Hallam, 1984). As this is contrary to the statigraphic evidence, Bailey & Stewart (1983) considered that, for an Earth undergoing expansion with time, the bulk of the oceans would have to be outgassed since the Palaeozoic, requiring fundamental changes in atmosphere, climate, biology, sedimentology and volcanology.

Palaeomagnetism has long been considered the cornerstone of the theory of plate tectonics (Butler, 1992), supplying data about past locations of continents and ocean plates, providing evidence about motion histories of suspect terranes, continental growth and mountain belt formation, and the Earth's palaeoradius. The published analysis of palaeoradius by van Andel & Hospers (1968a, 1968b, 1968c) and McElhinny et al (1978) set out to demonstrate that, within the limits of confidence, theses of exponential expansion or moderate expansion of the Earth at the expense of oceanic lithospheric accretion is contradicted by the palaeomagnetic data (Khramov, 1987). This therefore led palaeomagneticians to conclude that there has been no significant change in the palaeoradius of the Earth with time.

As previously mentioned, recent literature indicates that there is an increasing awareness of these "perceived problems" confronting conventional plate tectonics, modeled on a static Earth radius. Empirical small Earth modeling detailed in the previous section suggests that the present concepts of plate tectonics- continental drift- polar wandering may indeed need to be re-evaluated, revised, or rejected as Smiley (1992) indicated. With this in mind the remaining section will be devoted to a brief consideration of the relief of lithospheric curvature, orogenesis, accumulation of the hydrosphere and atmosphere, and a critical study of palaeomagnetism under conditions of exponential Earth expansion with time.

Back to Contents

Relief of lithospheric curvature

The relief of surface curvature during Earth expansion is demonstrated empirically on the small Earth models shown in Figures 24, 25, 26, 27, 28, 29, 30, 31, 32 and 33. During construction of these small Earth models it was found increasingly necessary to adjust continental margins, and rotate continents and/or cratons in accordance with the magnetic isochron data to account for the changing surface curvature with time.

The relief of lithospheric curvature on an Earth undergoing progressive expansion with time was considered at length by Rickard (1969) and Dooley (1973, 1983), and stress relief breifly considered by Weijermars (1986). The later two authors discounting Earth expansion on the grounds of spatial incompatibility resulting from the changing surface curvature. This spatial incompatibility was emphasised by Jeffreys (1962), in commenting on Barnett's (1962) small Earth models (Figures 4 & 5), pointing out that areas on Barnett's 4½ inch diameter sphere cannot be placed on his 3 inch diameter sphere without undue distortion. The reconstruction of continents on a small Earth was therefore considered by Jeffreys (1962) to be dependant on the distribution of continental distortion.

Dennis (1962), in reply to Jeffreys (1962), suggested however that the implied lithospheric distortion could be met because pseudo-viscosity of the crust and distortion by shear would lead one to expect continental distortion in any event, and further considered that the outline of continental margins may have changed in time by accretion of the continents, by loss of continental crust, or both.

This spherical distortion was considered by van Hilten (1963, 1965), during early investigations into palaeomagnetism, suggesting that, during Earth expansion, the outer rim of the continental lithosphere would distort and be displaced by forming a number of radial tears. Van Hilten (1963) introduced the term "orange peel effect" (Figure 34) to describe such a process. This early "orange peel" model, which suggests that the continental outlines are formed by merely "pumping up" the size of the Earth and splitting the continental lithosphere, has however never been seriously accepted (Owen, 1983b). It was suggested instead by Carey (1963) that the greater separation of the southern continents, and general northward migration of all continents, has resulted because of a greater expansion of the southern hemisphere than in the northern hemisphere.

Figure 34 Van Hilton’s (1963) "orange peal effect" model for Earth expansion. Van Hilton suggested that, during expansion of the Earth from A to B, the continental lithosphere would distort and displace by forming radial tears. (From Owen, 1983b)

The relief of surface curvature on expansion of a cratonic sector, settling from a smaller radius to fit an expanding globe, was discussed at length by Rickard (1969), suggesting a possible mechanism for "geosynclinal" formation and orogenesis. In contrast to earlier assumptions that continental plates adjust to the new curvature during expansion by plastic flow and cracking (eg. van Hilten, 1963; Creer, 1965), Rickard (1969) suggested that the Earth's crust would be sufficiently strong enough during expansion to require a considerable time lag before complete adjustment of continental curvature was achieved (Figure 35). Rickard argued that, during an expansion of the Earth, "geosynclines" would initiate as furrows along the margins of continental cratons because of the differential "radial expansion" during expansion of the oceanic lithosphere. The effective increase of continental craton slope by a few degrees would therefore increase the rate of sedimentation, giving rise to a rapid accumulation of thick sedimentary piles.

Figure 35 Rickard’s (1969) model for relief of surface curvature during Earth expansion. Figure A represents an "initial stage" of "marginal geosynclinal furrow’ development; Figure B represents a "critical stage" in the development of orogenesis, rifting and initiation of sea-floor spreading and; Figure C represents the "relieved stage" of curvature readjustment, isostatic uplift, block faulting, sea-floor spreading, continental separation and development of island arcs. (From Rickard, 1969)

The delicate state of balance between the opposing forces involved in curvature relief was therefore considered by Rickard (1969) to account for the complex variety of vertical movements during early "geosynclinal" activity, and eventually a critical stage would be reached when magmatic activity and rising geo-isotherms caused orogenesis.

Carey (1975; 1983a) was critical of Rickard's (1969) model because of his assumption of a significant enduring strength in the continental crust, and super-elevation of the central sector of the craton. Carey (1975) considered that, because of the rapid adjustment of isostatic inequalities in the asthenosphere the required super-elevation could never come about. Carey appreciated that the central sector must rise "because of the megatumour beneath it", however maintained that, it would never depart far from isostatic equilibrium, nor would there be any lateral gravitational force beyond that arising from hydrostatic equilibrium.

Carey (1976, 1986) considered instead that "the Earth consists of a crystalline mantle some 3000 kilometres thick over a fluid core", the first result of expansion would be the rupture of the whole mantle into polygonal blocks of a few 1000 kilometres across (Figure 36), surrounded by accreted oceanic crust added during the last 160 million years. These primary polygons would then be patterned by second-order polygonal basins and swells (Figure 37) extending through both the continental and developing oceanic lithosphere.

Figure 36 Lithospheric first-order primary polygons surrounded by circum-continental spreading "diapirs". Each numbered primary polygon consists of a continent surrounded by its accreted oceanic crust, added during the past 200 million years. (From Carey, 1983c)

As the Earth continued to expand the first adjustment to a decreasing surface curvature would occur at the primary spreading ridges, extending to the second-rank basins and swells as the lithosphere isostatically adjusted. Further adjustment would then continue down through a hierarchy of fractures and ultimately to ordinary joints (Carey 1975), in consequence to final adjustment of the changing curvature.

Figure 37 Second-order basin-and-swell polygonal patterns developed throughout both continental and oceanic lithosphere. The patterns are inferred to represent lithospheric adjustment resulting from relief of surface curvature. (From Carey 1986)

Within the context of Global Expansion Tectonics, when considering the mechanism for relief of lithospheric distortion during progressive Earth expansion, it is important to recognize that, geophysically and geologically, continental lithosphere is made up of a broad tectonic distribution of cratons, orogens, and sedimentary basins. Each of these have their own definitional tectonic framework, which differ fundamentally from the modern ocean basin lithosphere (Owen 1992). Previous authors do not address this tectonic framework, considering instead that continental lithosphere has acted as either rigid plates (Dooley, 1973, 1983), hence the continental plates would tend to retain the curvature of an earlier smaller Earth, or that a large majority of the continental crust was already in existence since Archaean times (Weijermars, 1986), hence the growth of continental crust through geological time was considered negligible.

An inspection of continental geological maps of the world (eg. Derry, 1980; Larson et al, 1985; CGMW & UNESCO, 1990) demonstrates this tectonic and chronological hierarchy of continental lithosphere on a global scale, and similarly the oceanic isochron data of Larson et al (1985) and CGMW & UNESCO (1990) demonstrates the broad chronological hierarchy of the ocean basins. From the continental data of Derry (1980), Larson et al (1985), and CGMW & UNESCO (1990) it can be seen that, although highly variable in size and shape, exposed Archaean cratonic regions commonly attain dimensions of 1000 kilometres to 3000 kilometres in any one direction. These Archaean cratonic regions are then further subdivisible into provinces or sub-provinces attaining dimensions of 500 kilometres to 1000 kilometres. These accord with Carey's (1975) first and second order polygonal hierarchy respectively.

Figure 38 Cross section of the Earth demonstrating the magnitude of change in surface curvature required during Earth expansion from Early Jurassic to the Present. Mid-point super elevation for the 1000 kilometre diameter craton shown amounts to approximately 17.5 kilometres of vertical adjustment and/or erosion during equilibration to the present surface curvature.

For a hypothetical Archaean craton of say 1000 kilometres diameter, which has remained tectonically stable during Earth expansion, the calculated difference in mid-point elevation between the surface curvature on a sphere of 53% palaeoradius and surface curvature of a sphere of present day radius amounts to approximately 17.5 kilometres, and for a 500 kilometres diameter craton, 4.5 kilometres (Figure 38). These orders of magnitude are within the realms of observable erosion and planation of these cratons. These values would be less if Carey's (1975) third, fourth and fifth order hierarchy of fractures are considered, in consequence to final adjustment of the changing surface curvature. Similarly, for the same Archaean cratons, the amount of peripheral extension required during equilibration of surface curvature to the present Earth radius amounts to approximately 8.3 kilometres and 1.0 kilometres for the 1000 kilometres diameter and 500 kilometres diameter cratons respectively. These equate to between 2.6 and 0.6 metres of peripheral extension per kilometre.

For an intra-cratonic orogen or basinal region, where crustal stability is not a definitional requirement, a hypothetical cross-section of a continent is considered in Figure 39. In this example two cratons are exposed, separated by an intra-cratonic sedimentary basin, with a total chord length of say 4000 kilometres. Each tectonic unit represents approximately one third of the primary 53% palaeoradius continental fragment, and both cratons are dimensionally stable. Assuming a constant chord length, the calculated foreshortening within the intra-cratonic sedimentary basin during crustal isostatic equilibration to the present day surface curvature therefore amounts to approximately 190 kilometres. This again is in the right order of magnitude for observed crustal foreshortening and potential uplift within existing orogenic regions.

Figure 39 Intracratonic basin foreshortening during asymmetric expansion of the Earth from Early Jurassic to the Present. In the example shown, basin foreshortening amounts to approximately 190 kilometres, giving rise to diapiric "compressional" type orogenesis.

In contrast, for oceanic lithosphere, it must be emphasised that preservation of oceanic lithosphere during Earth expansion is considered cumulative with time, and therefore preservation occurs at a progressively increasing Earth radii. This is shown schematically in Figure 40, where continental cratonic plus marginal basinal or orogenic sedimentary continental lithosphere is bounded on both sides by a symmetric accumulation of oceanic lithosphere. The oceanic lithosphere shown in the figure has accumulated during chron intervals of equal duration, from an initial 53% palaeoradius to the present, and demonstrates the profile which would be preserved if no oceanic isostatic equilibration occurred. The amount of oceanic lithospheric distortion required to maintain isostatic equilibration would simply involve ridge-parallel stretching and faulting, or ridge-transverse fracturing, all of which are well documented features of the modern ocean basins (eg. Meyerhoff et al, 1992).

Figure 40 Cross section of the Earth showing relief of surface curvature of continental and oceanic lithosphere, from Early Jurassic to the Present.Oceanic lithosphere is shown accumulating under conditions of increasing palaeoradius with time, with no adjustments made for progressive relief of surface curvature. Isostatic adjustment of surface curvature at the continent/oceanic lithospheric boundary results in trench/underpinning, or extensional basin settings depending on the stress règime present.

Back to Contents

Orogenesis

When Vogel (1983) enclosed a small Earth model of 55% palaeoradius inside a transparent plastic sphere representing the present Earth radius he concluded that, in general, the continents "moved out radially from their Precambrian positions to reach their modern positions" (Figure 7). Brunnschweiler (1983), as previously mentioned, considered however that, if Earth expansion is essentially a radial movement then its tangential plate displacements are only apparent, not real. Radial expansion on its own would therefore prevent rather than create tangential crustal movements of the sort which build mighty orogenic belts, the inner structure of which Brunnschweiler (1983) considered pointed to a horizontal foreshortening through collision.

It is unfortunate that this "radial expansion" concept has crept into the published literature. As Carey (1963) first recognised, and Barnett (1962, 1969) demonstrated using small Earth models, the present Earth has a hemihedral asymmetry, with an antipodal distribution of continents and oceans (Figure 5). What this implies is that the southern continents have separated much greater distances than those of the northern hemisphere, with a much greater insertion of new oceanic lithosphere in the southern hemisphere. This distribution of continents and oceans suggests that the Earth expansion process is asymmetric rather than radial, and therefore plate motion is made up of both tangential and radial vector components.

This antipodal distribution and asymmetric expansion process giving rise to both tangential and radial vector components is empirically confirmed by small Earth modeling (Figures 24, 25, 26, 27, 28, 29, 30, 31, 32 and 33). This process, in conjunction with relief of surface curvature, is considered to be the primary mechanism for continent to continent (or craton to craton) interaction during exponential expansion, resulting in orogenesis.

Figure 41 Rickard’s (1969) model for development of a "geosynclinal trough" prior to orogenesis. Figure A represents a "critical stage" of development where tangential shear is balanced by the strength of the crust and downward acting weight of the sediments and; Figure B represents orogenesis, where terminal tectogenesis is induced by a rise in the geo-isotherms and granite magma. (From Rickard, 1969)

Compression giving rise to orogenesis during Earth expansion was considered by Rickard as early as 1969. The compression was considered to have resulted from basin inversion and by interaction of the continental plate as the basin adjusted its curvature (Figure 41). A lateral outwards movement of the continental plate then caused compressional buckling and overthrusting of the "sedimentary fill" within a "narrow geosynclinal trough". Similarly, intracontinental "geosynclines" were also considered to have developed where continental plates were fractured internally, giving rise to considerable lateral compression as the two adjacent plates moved together during relief of surface curvature.

Rickard's (1969) model for "geosynclinal" development however implied that Earth expansion is essentially a radial process (Figure 35), and consideration of Figure 41 also implies that orogenesis is a post-Early Jurassic, post-sea floor spreading phenomena. As shown by the Global Expansion Tectonics small Earth models however, pre-Early Jurassic continental lithosphere completely enclosed the Earth as a single supercontinent, with ocean basins confined to shallow intracratonic seas.

The model for "geosynclinal" development and orogenesis put forward by Carey (1975, 1976, 1983a, 1986,1994) for an expanding Earth undergoing continuous crustal extension is shown in Figure 42.

Figure 42 Carey’s (1994) model of diapiric orogenesis for a symmetrical single phase orogen rising about 100 kilometres. The top figure shows initiation of primary stretching in the continental crust leading to "necking" or thinning. The bottom of the crust and mantle diapir below it rise, and continues to do so during orogenesis. The middle figures show two contrasting sites of sediment deposition, developing as a deep axial eugeosyncline and shallow marginal miogeosynclines. As regional isostatic equilibration is approached, a positive gravity anomaly over the ridge is balanced by a negative anomaly over the axial zone. The lower figures show a continuing and accelerating accent of the deep diapir, signalling the onset of orogenesis and nappe-like overthrusting of the eugeosynclineal sediments, as a result of crustal tension of a few tens of kilometres. (From Carey, 1994)

In Carey's model, the development of a simple diapiric orogen results from initiation of primary stretching in the continental crust due to "radial" expansion, leading to "necking" or thinning. The top and bottom surfaces of the continental crust converge towards zero with time at some 5 kilometres below sea-level, during which time the mantle rises some 30 kilometres. Thus, although the surface of the thinning continental crust subsides steadily, the bottom and the mantle diapir below it rises (Carey 1975; Tanner 1983b), continuing to do so throughout orogenesis. Carey (1975) considered that crustal thinning, caused by the expanding interior, resulting in gravity drive (eg. Ramberg, 1983) towards isostatic equilibrium, causes all the motions necessary for orogenesis.

In contrast, the classical plate tectonic theory incorporates two profoundly different explanations for orogeny, noncollisional (Cordilleran) and collisional (Cebull & Shurbet, 1992). The noncollisional model requires an ongoing and comparatively continuous event, subduction, to produce a discontinuous event, orogeny. Cebull & Shurbet (1992) considered that the model itself contains no mechanism by which the continuous-to-discontinuous transformation is accomplished and because of this, and failure to express a means of transmitting the stresses required for orogenic deformation into the core of the orogen, the noncollisional model is presently little used in the interpretation of ancient orogenic belts (Cebull & Shurbet, 1992).

The plate tectonic collisional model explains most structures in orogenesis in terms of lateral shortening through primary horizontal collisional compression and, in addition, is used to explain how subduction can be continuous and orogeny discontinuous. The collisional model is also used to predict the occurrence of exotic terranes (Cebull & Shurbet, 1992). Cebull & Shurbet, (1992) concluded however that neither model in the two-fold explanation for orogeny depicted in conventional plate tectonic theory is sufficiently comprehensive to explain the apparent complexities of orogenesis and, moreover, that both together are inadequate.

Orthodox plate tectonic compressional theory however agrees that during the "geosynclinal" stage of orogenesis the continental crust must thin, otherwise there is no possibility of maintaining even approximate isostatic balance through the millions of years involved in this stage (Carey 1986). Orthodox tectonics then reverses from crustal extension to crustal shortening (Carey 1986) to produce orogenesis. By contrast, in Carey's (1994) expansion model, extension persists through all stages, and the gravity-driven sub-crustal diapiric motion depicted in this model (Figure 42) is upward at all times.

Small Earth modeling, while not of a sufficient scale to model orogenesis in detail, suggests that orogenesis is more complex than depicted in all of the models above, with every gradation from compressional to translational and torsional stress regimes involved. In general, the Global Expansion Tectonic small Earth models indicate that orogenesis results from continent to continent (or craton to craton) interaction, either compressional or translational, as a direct result of asymmetric radial expansion. The tangential vector component of this asymmetric process providing the means of transmitting the continuous stresses required for orogenic deformation, and also the means for discontinuous and/or intermittent orogeny.

It is considered that the spherical geometry involved during relief of surface curvature and crustal fragmentation provides the mechanism for basin extension, "geosynclinal sedimentation and orogenesis. Once the enduring strength of the continental crust is overcome by gravity, the super-elevation of the central sector of the craton then progressively subsides causing diapiric uplift and orogenesis along the margins of the craton.

Back to Contents

Hydrosphere and Atmosphere Accumulation

Fundamental to the concept of Global Expansion Tectonics is the premise that ocean water and atmospheric accumulation has been continuous throughout much of geologic time. As the generation of oceanic lithosphere depends fundamentally on the same process as the outgassing of juvenile water, Carey (1994) considered it would be expected that, the volume of sea-water and the capacity of the ocean basins both increase in a related way, but not necessarily in phase.

Current theories on the formation of the hydrosphere and atmosphere fall into two main categories (Bailey & Stewart, 1983; Jackson & Pollack, 1987) namely:

1. a massive early outgassing from the Earth (eg. Fanale, 1971)

2. a gradual evolutionary accumulation through continued volcanic activity (eg. Cloud, 1968; Anderson, 1975; Rubey, 1975)

3. while Global Expansion Tectonics requires an accelerating accumulation late in the Earth's geological history

If lithospheric development has accelerated with time as is suggested, it is logical to conclude that (2) and (3) are the same process. This is in keeping with observations of the development of oceans such as the Arctic, Atlantic, and Indian, which date from the Mesozoic and have doubled their area since the Eocene (Carey, 1975), and also the Pacific Ocean which small Earth modeling suggests was a fraction of its present size before the Mesozoic (eg. Meservey, 1969; Avias, 1977; Shields, 1979, 1983b, 1990; Crawford, 1986). Similarly, proportions of exposed continental igneous, metamorphic and sedimentary rocks suggested to Blatt & Jones (1975) that the relationship between geological age and outcrop area increases lognormally with time. This indicated that, while there were extensive seas older than the Mesozoic, oceans of the modern type are a new phenomena.

Rubey (1975) suggested that, the whole of the waters of the oceans have been exhaled from the interior of the Earth, not as a primordial process, but slowly, progressively and continuously throughout geological time. Carey (1988) similarly concluded that, as the generation of the ocean floors depends fundamentally on the outgassing of juvenile water, it would therefore be expected that the volume of sea-water (and atmospheric gases) and capacity of the ocean basins both increased, but not necessarily precisely in phase, in a related way.

Studies of melts of igneous rocks (eg. Anderson, 1975; Wyllie, 1979; Jackson & Pollark, 1987; Menzies & Hawkesworth, 1987) indicate that the solubility of H₂O increases with increasing pressure until a maximum value is reached in the mantle. Middlemost (1985) quoted examples of 21.0 wt% H₂O for a rhyolitic melt and 14 wt% H₂O for a basaltic melt at a pressure of 1.0 GPa and a temperature between 1000 to 1200°C. At higher pressures the solubility of H₂O in ultramafic rocks is also very high with diopside and fosterite dissolving over 20 wt% H₂O above 2.0 GPa. For silicate magmas Middlemost (1985) indicated that CO₂ is generally considered to have a low solubility at low pressures however above 1.5 GPa significant amounts may be dissolved, for example 9.0 wt% CO₂ in olivine bearing nephelinite melts at 3.0 GPa. Middlemost (1985) concluded that if H₂O and CO₂ were available, they should both be highly soluble in the magmas normally generated in the upper mantle.

Eggler (1987) considered that the volatile species in the system C-O-H-S could exist in the Earth's mantle in volatile bearing minerals. These could be dissolved in silicate- or carbonate-rich melts, in a separate supercritical fluid, or possibly in a dense silicate-volatile fluid at pressures exceeding a second critical end-point. It was also considered possible (Eggler, 1987) that solution of volatiles in crystalline minerals represent a significant repository for volatiles (eg. Aines & Rossman, 1984). These volatile-bearing minerals include amphibolite and phlogopite (H₂O), carbonates (CO₂), and sulphides (S), although other minerals such as hydrated magnesium silicates were also considered important in some situations. All the possible volatile species (H₂O, CO₂, CO, CH₄, H₂, SO₂ and H₂S) are soluble in silicate melts (Eggler, 1987), with H₂O and CH₄ being more soluble than CO, CO₂ and H₂S (Mysen et al, 1976; Mysen, 1977; Holloway, 1977; Eggler et al, 1979; Eggler & Baker, 1982).

Menzies et al (1987) in a study of metasomatic and enrichment processes in lithospheric peridotites and its effect on the asthenosphere-lithosphere interaction concluded that relatively high-temperature styles of metasomatism in the upper mantle is characterised by silicate melt (Fe-Ti rich) metasomatism whose chemistry is controlled by the presence and migration of silicate melts and the stability of amphibole, and is generally associated with regions of tectonic activity, crustal thinning and elevated heat flow. This elevated heat flow was further considered to be consistent with the presence of high-temperature silicate melts in the upper mantle.

The progressive depletion of volatiles, such as H₂O and CO₂, from the Earth's mantle significantly reduces the creep strength and melting temperatures in these silicates, which Jackson & Pollack (1987) considered may be accompanied by an evolving rheology with increasing viscosity and less efficient heat transfer with time..

It is considered that this reduction of creep strength and melting temperature is intimately related to the changing core-mantle P-T-g conditions during expansion of the Earth with time and that depletion of volatiles from the mantle may therefore be a natural consequence of the changing rheology. It is logical to conclude therefore that if the T-P-g conditions were high during the pre-Early Jurassic, as indicated by Global Expansion Tectonics, then volatiles could have existed within the mantle until conditions were no longer suitable for their retention.

Back to Contents

Palaeomagnetism

Palaeomagnetism is defined (Piper 1989) as the study of the fossil remanant magnetism residing in rocks. The application of palaeomagnetism to the rock record falls essentially into two parts; data applicable to post-Mesozoic/Cenozoic times younger than about 205 million years and; data applicable to pre-Mesozoic times.

Post-Mesozoic palaeomagnetic data are currently used to place quantitative constraints on the age and spreading history of modern oceanic crustal regions and relative motions of continental crust. They also define the polarity history of the magnetic field, and the palaeomagnetic record is used in the investigation of the nature of the geomagnetic field with time. Pre-Mesozoic palaeomagnetic applications are restricted to studies of relative motions of the continental crust, since no oceanic crust older than about 205 million years exists today. The application of palaeomagnetism to these increasingly older rocks is generally considered important in plate tectonics in defining the relative motions of continental crustal fragments. The palaeomagnetic data, applicable to the Early Jurassic crustal break-up and pre-breakup Palaeozoic intracratonic basinal sedimentary phase, is currently being extended to include the Proterozoic, and ultimately in constraining the Archaean crustal assemblage.

The principles and techniques of palaeomagnetism are described in detail in standard texts by Irving (1964), McElhinny (1973), Merrill & McElhinny (1983), Tarling (1983), Jacobs (1987), Piper (1989), Butler (1992) and van der Voo (1993), and reviews by Cox & Doell (1960) and Creer (1970). Conventional palaeomagnetic equations cited in this paper are based on a geocentric axial dipole model and detailed in Stacey (1977) and Butler (1992). These equations are used to determine palaeopole positions and statistically analyze palaeomagnetic data on the present sized Earth.

Back to Contents

Application of palaeomagnetic data

Within this paper the collection and statistical treatment of palaeomagnetic site data is acknowledged to have reached a high degree of precision. It is emphasised that the fundamental premises of palaeomagnetism are not contradicted by Global Expansion Tectonics. However, because conventional palaeomagnetic equations are intolerant of any change in Earth radius, the application of this site data, and conclusions drawn from the results, are considered erroneous. The application of palaeomagnetic data beyond this brief introduction requires a considerable input from a dedicated, but sympathetic palaeomagnetician.

Conventional palaeomagnetic equations, used in the application of palaeomagnetism to determine pole positions, are based on an Earth with a palaeoradius equal to, or approximately equal to the present radius. It should be realised that no provision is made in these equations for the effects of any potential change in palaeoradius with time. These equations simply measure the angle subtended by the palaeopole and are plotted as angular distances from the site to the palaeopole (Carey, 1994). Any variation in palaeoradius cannot be accommodated in these equations as they currently stand.

Depending on the site data and method used, estimations of palaeoradius using palaeomagnetism have been published which vary from fast expansion rates (van Hilten, 1963; Ahmad, 1988a), compatible with expansion rates derived from empirical small Earth modeling (e.g. Hilgenberg, 1933; Carey, 1958; Vogel, 1983), to slow or negligible expansion rates (Cox & Doell, 1961a, 1961b; Ward, 1963; Hospers & van Andel, 1967; McElhinny & Brock, 1975; McElhinny et al, 1978), (e.g. Figure 20). With this in mind, it is intended to deal with the application of palaeomagnetism to Global Expansion Tectonics by first reconsidering the dipole equation, in view of an exponential increase in palaeoradius, and apply this to the determination of pole positions from site mean data. This will be followed by a series of examples demonstrating the effects that exponential Earth expansion have on calculated palaeomagnetic data, prior to dealing with estimation of palaeoradius.

Back to Contents

The "Dipole Equation"

The "dipole equation" is the most fundamental equation used in conventional palaeomagnetics, and is given as:
 

Where I is the mean inclination of the magnetic field determined from site data; L (Lambda symbol in Figure 43) is the latitude, and p is the colatitude determined from I (Figure 43).

Figure 43 Geocentric axial dipole model. A magnetic dipole M is located at the centre of the Earth and aligned with the rotation axis. The geographic latitude is defined by l, the mean Earth radius r_e, the magnetic field directions at the Earth’s surface produced by the geocentric axial dipole are schematically shown, inclination I is shown for one location and N is the north geographic pole. (From Butler, 1992)

Rearranging the dipole equation gives the colatitude p as:
 

This equation represents a measure of the great-circle angular distance from the mean sample site to the magnetic pole and, like latitude, is independent of any radial or time constraints imposed by the sample site. The dipole equation is in fact a general equation applicable to any sized magnetic sphere which obeys the geocentric axial dipole model outlined, be it a spherical hand held pocket magnet, or a planet. The magnetic lines of force behave in exactly the same manner, irrespective of scale, and for a given site inclination the colatitude (or latitude), calculated from the dipole equation, will always remain the same.

Figure 44 demonstrates this very important characteristic of the dipole equation whereby, for a given site value I, the dipole equation remains true for an infinite number of sites along a radius vector R, passing through the centre of the Earth to the site location and beyond. What must be realized, and is fundamental to the estimation of palaeoradius, is that colatitude, calculated from an inclination I at sites S₁.....S_n, located along the radius vector R, is equal to a constant angular measurement p. At site S₁ this colatitude p however represents an arcuate distance D₁ which is not equal to distances D₂.....D_n for sites S₂.....S_nusing the identical values of inclination I and colatitude p.

For a simplistic radial expansion of the Earth from R₁ to R₂.....R_n the palaeopole positions P₁, P₂.....P_n calculated from the conventional dipole equation are shown in Figure 44. Because the conventional dipole equation uses angular measurements, and has no provision for either a radial or time component to compensate for the shift in actual palaeopole position with expansion, the calculated pole positions will always coincide with the geomagnetic pole N.

Figure 44 Cross section of a number of geocentric axial dipolar magnetic spheres demonstrating the characteristics of the conventional dipole equation. For a given site value I the conventional palaeopole positions P₁ to P_n for sites S₁ to S_n coincide with the geomagnetic pole N. The actual palaeopole positions defined by the arcuate distances D₁ to D_n are shown for comparison.

In order to determine the ancient palaeopole position P_a on the present day Earth, with palaeoradius varying exponentially with time, consider Figure 45. At site S₀ located at the present radius R₀, using the conventional palaeocolatitude equation, an inclination I gives a colatitude p which equates with the palaeocolatitude locked into the rock record at site S_a and palaeoradius R_a.

Figure 45 Cross section of a number of geocentric axial dipolar magnetic spheres used to determine the actual palaeopole position P_a from palaeomagnetic site data located at site S₀on the present day Earth of radius R₀.

At site S_a the arc distance D_a is equal to:
 

Where p is in radians, and rearranging:

To determine the palaeopole position P_a located on the present radius Earth, which equates to the ancient palaeopole position P_adetermined from site S_a:

The mathematical relationship for an exponential increase in the Earth's palaeoradius, derived from empirical measurements of oceanic and continental surface area data was previously found to be:
 

Where: R_a = ancient palaeoradius of the Earth, R₀ = present radius of the Earth, R_p = primordial Earth radius = approx. 1700 km [1800 km], e = exponential, t = time before Present (negative), k = a constant = 4.5366 x 10^-9/yr

Incorporating Equation 1 for R_a gives:
 

And incorporating Equation 2 for p gives:
 

For any site sample, constrained by the age of the rock sequence containing the site data, the palaeocolatitude from site to the ancient palaeopole position on an Earth of present radius is therefore equal to:
 

At this point it should be reiterated that the palaeocolatitude values determined using the conventional dipole equation remain true for any radius sphere, and is independant of both time and palaeoradius. The modified equation above is however neccessary, in order to convert the ancient geographical location on an Earth of reduced palaeoradius, to the modern, Present day geographical grid dimensions. The relative geographical location, determined using the conventional dipole equation remains true regardless of palaeoradius. The application of this "modified dipole equation" to palaeomagnetic site data enables the ancient palaeocolatitude, determined from site mean data existing at the time the site samples were locked into the rock-record, to be simply converted to the present geographical grid system. The use of this equation then enables the ancient palaoepole position to be correctly determed on the present Earth radius.  Equation 3 thus forms the basic "modified dipole equation" for Global Expansion Tectonics, which will now be used to determine the ancient palaeopole coordinates from site mean data located on an Earth of the present size.

Back to Contents

Determination of Pole Position

Site mean data determined from a set of site data are assumed by palaeomagneticians to represent a time-averaged field which compensates for any secular variation caused by non-dipole components (Tarling, 1971). For a geocentric axial dipole field the time-averaged inclination I, determined from site data, corresponds to the palaeocolatitude existing at the site when the site data were locked into the rock-record, and the time-average declination D indicates the direction, along a palaeomeridian, to the palaeopole. Calculation of the position of the palaeomagnetic pole on the present Earth surface is therefore a navigational problem in spherical trigonometry which uses the dipole equation (Equation 2) to determine the distance travelled from the observing locality to the pole position (Figure 46).
 

Figure 46 Determination of a magnetic pole from a magnetic field direction using the conventional dipole equation. Orthorhombic projection with latitude and longitude grid in 30° increments. Figure a: the site latitude and longitude is (L_s, F_s); the palaeomagnetic pole is located at (L_p, F_p), site colatitude is p_s; colatitude of the magnetic pole is p_p; and the longitudinal difference between the magnetic pole and site is B, Figure b: illustrates the ambiguity in magnetic pole longitude. The pole may be at either (L_p, F_p) or (L_p, F’_p); the longitude at F_s+ #/2 is shown by the heavy line. (From Butler, 1992).

Details of the derivation of the following equations are given in Butler (1992). Sign conventions and symbols for geographic locations are as follows, adopted from Butler (1992): (Note: because of the difficulty of using Greek symbols as per the figures I have adopted L for the symbol Lambda, B for Beta, F for Phi, A for Alpha, and # for Pi)

• latitudes increase from -90° at the south geographic pole to 0° at the equator and +90° at the north geographic pole;

• longitudes east of the Greenwich meridian are positive, while westerly longitudes are negative and;

• (L_p, F_p) is the pole position calculated from a site-mean direction (I_m, D_m) measured at site location (L_s, F_s).

The pole latitude derived from spherical trigonometry is given by Butler (1992), (Figure 46) as:

The longitudinal difference between pole and site, denoted by b , is positive towards the east, negative towards the west, and is given by Butler (1992) as:
 

Where if:
 

Then the pole longitude:
 

But if:
 

Then the pole longitude:
 

For any site-mean direction (I_m, D_m) the associated circular confidence limit (a ₉₅) is transformed into an ellipse of confidence about the calculated pole position with semi-axes of angular length (d_p, d_m)given by Butler (1992), (Figure 47):
 

And:
 

Figure 47 The conventional ellipse of confidence about a magnetic pole position.

Orthorhombic projection. (From Butler, 1992)

These conventional palaeomagnetic equations however do not, and cannot acknowledge any variation in palaeocolatitude under conditions of a variable palaeoradius with time, as previously determined. What is assumed by these equations is that the palaeogeographical coordinate system indicated by the site-data is equivalent to the geographical coordinate system represented by the present site location. Using conventional palaeomagnetic equations to determine the pole position the two systems are therefore simply added, using spherical trigonometry, to give a pole latitude and longitude.

For an Earth undergoing an exponential increase in palaeoradius from the Archaean to the Present, the actual palaeocolatitude determined from site data was found to be:
 

Incorporating Equation 3 into the pole coordinate equations of Butler (1992) therefore gives equations, applicable to Global Expansion Tectonics, which determine the actual palaeopole positions on an Earth of present radius.

Palaeopole latitude therefore becomes:
 

Longitudinal difference:
 

Where if:
 

Then the palaeopole longitude:
 

But if:
 

Then the palaeopole longitude:
 

And ellipse of confidence:
 

And:
 

As previously mentioned, because these modified equations convert between geographical grids, the palaeocolatitude values determined are not representative of the ancient geographical location, the relative geographical location can only be determined using the conventional dipole equation. The application of these modified equations to site data on an Earth of present radius results in palaeopole positions which are closer to the present day site location than those determined using conventional equations. The north and south palaeopole positions are also not diametrically opposed as would normally be expected.

Back to Contents

Hypothetical Palaeomagnetic Pole Simulations

In order to demonstrate the effects of palaeomagnetic pole determination on an Earth undergoing exponential expansion, a number of simulated cases are presented below, prior to considering estimation of palaeoradius using palaeomagnetic data. This is considered necessary in order to fully appreciate a number of key palaeomagnetic phenomena unique to the Earth expansion process.

In the following examples it is assumed that the magnetic field was produced by a geocentric axial dipole, the spatial and temporal complications of any nondipolar fields are minimal, and the crustal fragments under consideration have re-equilibrated to the changing Earth curvature.

Hypothetical Example (1)

Consider a narrow equatorially aligned strip of continental crust containing palaeomagnetic site-data of Late Jurassic, chron M17, age (Figure 48), with an estimated palaeoradius of 4087.6 kilometres. The fragment of crust has been subjected to an asymmetric radial expansion of the Earth to the present radius and remains aligned along the equator without any fragmentation or distortion beyond an equilibration of the crust to the present surface curvature. The sample locations are spaced uniformly along the Late Jurassic crustal strip, and site-mean data for each locality all have a mean inclination I=0° and mean declination D=360°.

Figure 48 Palaeomagnetic pole simulation for an equatorially aligned crustal strip containing site data of Late Jurassic age. The crustal strip has been subjected to a radial expansion of the Earth, to the Present, without fragmentation or distortion beyond an equilibration to the present surface curvature. The figure shows north and south VGPs located along small circle arcs whose focal points correspond to the present day north and south GP.

From the modified dipole equations the calculated north and south virtual geomagnetic pole (VGP) positions are shown in Figure 48 as small circle arcs whose focal point coincides with the present day geomagnetic pole (GP) positions. The distance from the present day north and south GP to each VGP small circle arc being dependant on the age of the rock sequence under study, with younger rock sequences approaching the GP until they coincide at the present. On an Earth of present radius the north and south VGPs are also not diametrically opposed, the great circle separation being related to the palaeoradius existing at the time the site-sample NRM were acquired.

Using conventional dipole equations these same site data cluster as single north and south GPs as shown. In conventional plate tectonics a net north or south displacement or rotation of the crustal strip would therefore be reflected as a displacement of the GP positions, and displacement in time reflected as an apparent polar wander path (APWP)

Hypothetical example (2)

In the second example a similar narrow strip of Late Jurassic continental crust was aligned meridionally (Figure 49). The strip of crust was subjected to an identical asymmetric radial expansion to the present radius, and crustal equilibration to the present surface curvature, as in the previous example. In this example the strip retains a meridional alignment throughout, and equatorial site sample I=0 maintains an equatorial location. The sample locations are uniformly spaced, and site-mean data for each locality have mean inclinations I as shown, and mean declinations D=360°.

The north and south VGP positions, calculated using the modified dipole equations, are shown in Figure 49 as clustering as single north and south palaeomagnetic pole (PP) points with, in this example, latitudes coincident with the VGP small circles shown in Figure 48. For an asymmetric expansion involving a net north or south displacement of the crustal strip the VGP small circles would migrate north or south respectively, and similarly, a net rotation of the crustal strip will cause rotation of the small circles.

In contrast, the VGP positions calculated for the same site locations using conventional dipole equations are also shown (Figure 49). The conventional north and south VGPs are distributed along a meridional line located between the actual north and south PP positions, calculated from the modified dipole equations, and the present day north and south magnetic poles. The scatter of conventional VGPs along these north and south meridians increases away from the present day magnetic pole position, towards the actual PP position, as the site mean inclination increases.

Figure 49 Palaeomagnetic pole simulation for a meridionally aligned crustal strip containing site data of Late Jurassic age. The crustal strip has been subjected to a radial expansion of the Earth, to the Present, without fragmentation or distortion beyond an equilibration to the present surface curvature. The figure shows north and south VGPs clustering as north and south PPs located along a Late Jurassic small circle arc.

It can be seen from both of these hypothetical examples that, using the modified dipole equations to calculate pole positions for crustal fragments originating from an Earth of reduced palaeoradius, the actual PP positions will focus as a VGP small circle arc. The palaeolatitude of this small circle arc is dependant on the age of the rock sequence. Similarly north and south PPs located along the same great circle will not be diametrically opposed, their separation being a function of the palaeoradius existing at the time the NRM of the site sample was acquired.

The pole positions calculated from conventional dipole equations are, in contrast, scattered between the actual VGP small circle arc and, in these examples, the present day GP position. When both examples are combined it can be seen that the scatter of pole positions increases away from the GP towards the VGP small circle arc as the site mean inclination increases.

With an asymmetric Earth expansion where there has been a net northerly migration of the continental fragments for instance, VGPs determined from conventional dipole equations, while still scattered, will appear to form a tighter cluster, which is traditionally statistically treated to give a mean GP position for that particular geological age. In reality however, the equatorial VGPs will overshoot the calculated GP, depending on the net migration of the continental fragments, and the remainder will approach the GP.

Hypothetical example (3)

In order to demonstrate the extreme conditions presented in examples (1) and (2) above, on a more realistic continental scale, consider Figures 50 and 51. In these figures a 15° wide longitudinal strip (gore) of continental crust was taken from the same Late Jurassic sphere of palaeoradius 4087.6 kilometres. A present day 15° wide longitudinal strip is overlain in both figures for comparison.

The late Jurassic crustal strip has been subjected to the same asymmetric radial expansion to the present radius, and crust allowed to equilibrate in both an east-west and north-south direction to the present surface curvature, without fragmentation. In this example the Late Jurassic equator coincides with the present day equator, and the strip has retained its meridional alignment.

Mean inclinations shown were calculated from Late Jurassic palaeolatitudes at 15° intervals, and mean declinations D, for each site, were calculated as the angle between the present day meridian and the palaeomeridian. The site locations marked as small crosses in Figures 50 and 51 were clustered to form three subcontinents, designated A, B and C, ranging in size from approximately 1000 kilometres x 1500 kilometres to 1500 kilometres x 2000 kilometres. The VGP positions calculated using the modified pole equations are shown in Figure 50, and VGP positions calculated using the conventional pole equations are shown in Figure 51.

In Figure 50, although not shown at this scale, the calculated VGP positions form an elongate east-west cluster of poles coinciding approximately with the actual Late Jurassic PP position. The slight east-west VGP scatter and shortfall in tabulated PP longitude is within the limits of accuracy of the calculated data, and reflects a slight misrepresentation of the actual crustal distortion during surface equilibration to the present curvature.

The tabulated PP positions for each of the subcontinents and PP of the total VGPs shown in Figure 50 are however in close agreement, within the limitations of the data. The angular deviation from the actual Late Jurassic PP in all cases amounting to less than 12 kilometres, which confirms that all three continental fragments have retained their relative positions throughout the period of Earth expansion. Calculated palaeoradius R_a to present radius R ratios (detailed later) coincide for each subcontinent PP and total continental PP, which confirms that the palaeomagnetic site data originated from an Earth of much reduced palaeoradius.

Figure 50 Palaeomagnetic pole simulation for a 15° wide crustal strip containing site data, clustered to form three subcontinents, of Late Jurassic age. Dotted gore represents 15° of longitude on the Present Earth for comparison. The crustal strip has been subjected to a radial expansion of the Earth to the Present, without fragmentation or distortion beyond an equilibration to the present surface curvature. The figure shows the Late Jurassic pole position calculated using the modified dipole equations, and a tabulation of PPs and R_a/R ratios for each subcontinent and total area. The figure confirms that all three subcontinents have retained their relative positions throughout the period of Earth expansion.

In Figure 51 the VGP positions, calculated using conventional pole equations, for each of the site locations are shown as small circular markers and have been clustered into three groups, designated A', B' and C', corresponding to the subcontinents A, B and C respectively. While not shown in the figure the VGP positions increase away from the present day magnetic pole position, as the site mean inclination increases, as predicted. In addition, in this example the site locations and calculated VGP positions are located on opposite sides of the central meridian shown, reflecting the palaeopole overshoot as also previously mentioned.

Figure 51 Palaeomagnetic pole simulations for the same meridionally aligned 15° wide crustal strip as shown in Figure 50. The figure shows Late Jurassic pole positions calculated using conventional dipole equations, and tabulations of PPs and R_a/R ratios for each subcontinent and total area. VGPs derived from site samples are shown as small circle markers, circles of confidence are shown as large dotted circles, and dotted gore represents 15° of longitude on the present Earth for comparison. Conventional palaeomagnetic interpretation suggests that subcontinents B and C, for instance, are displaced terranes, or rafts of sialic crust, having migrated from the north to collide, forming a collage of accreted crustal fragments with the necessary destruction of intervening oceanic crust.

For each of the subcontinents shown in Figure 51 the calculated PP positions have separations in excess of 1100 kilometres and deviation from the actual Late Jurassic PP position varying from 877 kilometres for subcontinent C, to 3167 kilometres for subcontinent A. The actual scatter of VGP positions, shown by the circles of confidence for each subcontinental group, amounts to approximately 400 kilometres radius.

The clustering of site data into three subcontinents, although arbitrary, gives rise to three separate palaeomagnetic pole positions in Figure 51, and more if the subcontinental areas were smaller. Conventional palaeomagnetic practice would imply that subcontinents B and C, for instance, are displaced terranes or rafts of sialic crust, having migrated from the north to collide, forming a collage of accreted crustal fragments and the necessary destruction of intervening oceanic crust, some time in the past.

The difference being, in this hypothetical case, that the site data are known to have originated from a Late Jurassic small Earth with a palaeoradius some 64% of the present Earth radius and actual R_a/R ratio of 0.64. The R_a/R ratios calculated for each of the subcontinents shown vary from 0.73 to 0.97, with a mean value of 0.84 which, considering the wide scatter of VGP positions, in conventional palaeomagnetics is customarily taken as indicating no appreciable expansion.

Back to Contents

Estimation of Palaeoradius Using Palaeomagnetism

Interest in the application of palaeomagnetism to determine the Earth's palaeoradius was instigated by a suggestion of Egyed (1960) in support of his views on Earth expansion. The suggestion was taken up by Cox & Doell (1961a, 1961b) who used palaeomagnetic data from Western Europe and Siberia, and was quickly followed by Ward (1963) and van Hilten (1963, 1965) who, in addition, used new data from North America to determine the ancient palaeoradius for the Permian, Carboniferous, Triassic and Cretaceous periods. Similarly van Hilten (1968) introduced new data from South Africa and Antarctica, and McElhinny & Brock (1975) used palaeomagnetic data from Africa to estimate the Mesozoic palaeoradius.

Estimating the palaeoradius of the Earth using palaeomagnetic data was carried out by means of the following three calculation methods, summarised from van Andel & Hospers (1968a):

1. palaeomeridian method: used for calculations based on palaeomagnetic data situated approximately on the same palaeomeridian. The method was developed by Egyed (1960, first used by Cox & Doell (1961a) and later by van Hilten (1963);

2. triangulation method: used for calculations based on palaeomagnetic data situated on substantially different palaeomeridians. The method was originally developed by Egyed (1960) and used in a modified format by van Hilten (1963) and;

3. method of minimum scatter of virtual poles: developed by Ward (1963).

All of the above methods of estimating palaeoradius start with the same indispensable assumptions (van Hilten 1968), namely:

1. a dipole configuration of the ancient geomagnetic field;

2. the average direction of magnetisation of the investigated rocks of a certain age parallel the contemporary geomagnetic field and;

3. a constancy of area of the continents during the supposed contraction or expansion of the Earth.

Van Hilten (1968) considered that the first two assumptions are generally accepted in most studies of rock magnetism, while the third was based on the geological considerations accepted at the time that regarded the continents as relatively rigid slabs; as compared to the oceanic lithosphere and the Earth's mantle. Carey (1976, 1988) considered however that a continental block cannot simultaneously preserve total surface area, inter-site distances and inter-site angles whilst adjusting to the changing curvature of an expanding Earth. Think about it!

Egyed's Palaeomeridian Method

The palaeomeridian method was based on the assumption that the continents do not increase in area, hence the distance between any two points on a stable part of one continent remains constant. As the Earth's radius increases, the geocentric angle between the two points therefore decreases (Cox & Doell, 1961a).

Figure 52 Egyed's (1960) palaeomeridian method of determining palaeoradius of the Earth from palaeomagnetic site data located on the same palaeomeridian. At palaeoradius (a) inclinations I₁ and I₂ at sites 1 and 2 give palaeocolatitudes of T₁ and T₂  (T used for the symbol Theta) determined from the conventional dipole equation, and palaeopole position shown. Assuming constancy of continental surface area, after expansion to present radius (b) inclinations I1 and I2 at sites 1 and 2, and the distance between the sites remains the same. The palaeopoles indicated from the two sites are not the same and palaeoradius was determined as the one yeilding the tightest cluster of VGP positions. (From Clarke & Cook, 1983)

Cox & Doell (1961a) further considered that, if contemporaneous palaeomagnetic data from two localities on the same stable continental block have different inclinations I₁ and I₂(Figure 52) so that the two localities are on different circles of geomagnetic latitude, and if the ancient geomagnetic field was dipolar, then the ancient Earth radius R_a may be found from:
 

Where d is the linear length of the great circle arc connecting the two sampling sites (Hospers & van Andel, 1967; van Andel & Hospers, 1968a).

Cox & Doell (1961a) used this method to evaluate the Earth's radius during the Permian, from data analysed from palaeomagnetic studies of 16 sample sites from western Europe and 5 sample sites from Siberia (Figure 53). The European and Siberian data being chosen specifically because they were the only data available at the time located supposedly on, or approximately on, the same palaeomeridian.

Figure 53 Permian virtual geomagnetic pole positions used by Cox & Doell (1961) to determine the palaeoradius of the Earth. Palaeomagnetic data are from sampling sites located at (1) Siberia and (2) Western Europe, represented by virtual geomagnetic pole positions at (3) Siberia and (4) Western Europe. (From Cox & Doell, 1961)

The method of calculation adopted by Cox & Doell (1961a, 1961b) was to pair each of the inclinations from Western Europe with those from Siberia giving a total of 80 determinations of R_a. The average of the 80 values calculated for the Earth's radius during the Permian was found to be:
 

with a standard deviation of 1080 kilometres, and Ra/R ratio of 0.99 (van Andel & Hospers, 1968a).

Compared with the present Earth radius of 6371 kilometres Cox & Doell (1961a) therefore concluded that the Permian magnetic field, as seen from the two sampling areas almost 5000 kilometres apart on the European land-mass, was consistent with a Permian Earth radius equal to the present.

The palaeomeridian method of determining palaeoradius from site data located along the same palaeomeridian is mathematically sound only if the primary assumptions are correct. That is, assuming that the continental lithosphere between the sample sites has remained spatially static and site separation has remained essentially constant. For the excessive site separation chosen, and continental area involved however, Earth expansion must involve some adjustment for relief of surface curvature.

This point was considered by Carey (1958, 1975, 1976, 1986) and appreciated in principle by most palaeomagneticians considering palaeoradius (eg. Cox & Doell, 1961, 1961b; Ward, 1963; van Hilten, 1963, 1965, 1968; van Andel & Hospers, 1968a, 1968b; McElhinny & Brock, 1975; Schmidt & Clark, 1980) however was largely ignored.

Palaeomagnetic sample sites used by Cox & Doell (1961a) are shown in Figure 53 located in Western Europe (France and England) and Siberia, with a site separation of almost 5000 kilometres on the present radius Earth. This may be compared to the Arctic Ocean small Earth sequential spreading history (Figure 24) detailed previously. As can be seen in this small Earth figure, modeling indicates that the northern European continent has undergone a considerable amount of crustal extension, from the Early Jurassic to the present, in sympathy with opening of the Arctic Ocean. This extension occurred throughout the region, in particular the West Siberian Basin (Ob sphenochasm of Carey (1976)), the Baltic Sea and North Sea regions. The sample sites, when relocated on the Early Jurassic small Earth model also suggest that the actual site separation at that time, and throughout much of the Mesozoic, crossed the North Atlantic spreading axis, as it extended into the Arctic Ocean region.

Figure 54 Suggested radial dispersal of continental fragments and development of Tertiary intracratonic sedimentary basins for Western Europe and Siberia during the Phanerozoic. In this model geocentric angles p₁, p₂, and p₃ do not decrease as assumed by Cox & Doell (1961) hence site separation increases, resulting in a palaeopole position and R_a/R ratios which mistakenly suggest that the Earth has not expanded.

Figure 54 is a schematic representation of the northern European region used by Cox & Doell (1961a) demonstrating the effect of radial expansion, crustal thinning, fragmentation and formation of Tertiary intracontinental sedimentary basins. In this model the geocentric angles between sites do not decrease as assumed by Cox & Doell (1961a), palaeocolatitudes accord with the conventional dipole equation and site separation increases with time, resulting in Ra/R ratios approaching unity, mistakenly considered by Cox & Doell (1961a) to imply a constant Earth palaeoradius.

In contrast, by adopting the present day site separation of approximately 5000 kilometres, and assuming that the European continent has undergone a radial expansion of its continental lithosphere to the present, marked by opening of the Ob sphenochasm for instance, then during the Early Jurassic the site separation calculates as approximately 2650 kilometres. This equates with the actual separation of the same sites located on the Early Jurassic small Earth model (Figure 24).

Van Hilten's triangulation method

The method, developed by van Hilten (1963) and modified by van Hilten (1968), utilises palaeomagnetic data not located on the same palaeomeridian. The method uses spherical trigonometry to determine a pole position from the intersection point of great circles passing through the sample localities, in the direction given by the declination.
 

Figure 55 Van Hilton's (1963, 1968) triangulation method of determining palaeoradius of the Earth from palaeomagnetic site data not on the same palaeomeridian. Right figure shows Carboniferous and Triassic palaeomagnetic site and VGP data from North America and, left figure shows Permian palaeomagnetic data from Siberia, Russia and Western Europe. (From van Hilten, 1963)

Van Hilten (1963) used five sets of palaeomagnetic data of various ages from North America and Western Europe and initially concluded that the palaeomagnetic evidence seemed to indicate a noteworthy increase in the Earth's radius since the Carboniferous, the rate of which roughly agreed with the hypotheses of Carey (1958) and Heezen (1959). Van Hilten (1968) later modified his calculation methods to calculate the palaeomagnetic positions for different radii of the Earth, in order to determine the Earth's radius for which the coeval poles of one continent show the least scatter. The method was modified to make allowance for crustal deformation, although he concluded that the consistency of area of the continents during expansion or contraction seemed justified in first approximation.

In his revised estimates of palaeoradius for the Permian of Europe van Hilten (1968) maintained that there was an inconsistency in the data which may be explained by tectonic events. His remaining calculations for North America however failed to produce evidence for either a change in the Earth's radius or a constant one.

The triangulation method used by van Hilten (1963, 1968) acknowledges some degree of crustal deformation, however the method assumes that the angular relationships of the spherical triangles used on the present Earth's radius are consistent with those of the ancient palaeoradius. As Carey (1988) pointed out however, surfaces cannot be transferred between spherical surfaces of different radii without distortion of area, angle or shape.

The triangulation method of van Hilten is in effect demonstrated in hypothetical example (3) (Figures 50 and 51). Depending on the clustering and inclination of site data used in the investigation, the VGP positions will triangulate as a palaeomagnetic pole position. Ra/R ratios calculated from the PPs will vary from the correct ratio, derived from site data containing polar located site inclinations, to ratios approaching unity for site data containing equatorially located site inclinations. Because of the statistics used in reducing the scattered pole positions to a mean pole position, Ra/R values will always be higher than the true value, and figures of 0.73 to 0.97 calculated in the hypothetical example (Figure 51) are consistent with published values (eg. van Hilten, 1963, 1968).

Ward's method of minimum scatter of VGPs

The method of minimum scatter, developed by Ward (1963), was applied to Devonian, Permian and Triassic data from Europe and Siberia. The method was again based on the assumption that, during any change in the Earth's radius, the size of each continent remained constant, and during a particular geological age being studied, the position of the pole did not changed markedly.

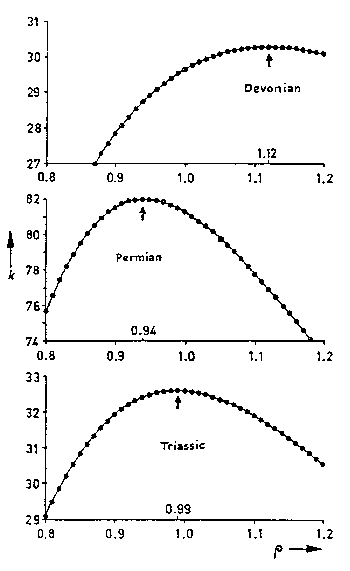
Figure 56 Ward's (1963) graphical representation of the dispersion of Triassic, Permian and Devonian palaeomagnetic data from Europe and Siberia. A pole precision parameter k is represented as a function of the hypothetical Earth's radius R_a, where R_a=1 for the Present Earth radius. Small arrows indicate the point on each graph where k is at a maximum, the point at which palaeomagnetic poles had minimum dispersion. (From Ward, 1963)

The method of minimum scatter of VGPs established the Earth's palaeoradius by generalizing from pairs to sets of site data, using the criterion that the most probable palaeoradius was the radius derived from a set of palaeomagnetic pole positions which had minimal dispersion (Ward 1963). The analysis of dispersion on a sphere was performed by Fisher's (1953) statistical method whereby the pole positions were assumed to be distributed about a true mean. The Fisher (1953) method involved determination of the dispersion of a population of directions, referred to as the precision parameter k, where the most probable ancient Earth radius was considered to be where k was maximum (Ward 1963).

The results of Wards determinations were presented as graphs of pole precision k as a function of the hypothetical Earth's radius, where radius equals 1 for the present radius (Figure 56). From these Ward (1963) concluded that the results for Europe and Siberia could not be considered to indicate any variation of the Earth's radius during the Devonian, Permian or Triassic periods from the present radius.

Irrespective of the lack of acknowledgment of any crustal distortion within the European continent, or the possibility of radial crustal expansion, the primary flaw in this method of palaeoradius determination is the assumption that the most likely palaeoradius is that at which the precision parameter k is maximum, hence VGP scatter is at a minimum.

Hypothetical examples (1) (Figure 48) and (2) (Figure 49) demonstrate two important features not considered by Ward (1963):

1. during Earth expansion ancient pole positions disperse along a small circle arc determined by the age of the rock formation, and;

2. pole positions, using conventional pole equations, actually reach a maximum dispersion when Ra = Ra and a minimum dispersion only when Ra approaches unity R, on an Earth of the present radius.

Ward's method of minimum scatter of VGPs was used by McElhinny & Brock (1975) for twenty six pole positions from Triassic and Cretaceous sample sites at widely separated parts of Africa (Figure 57). The mean poles determined by McElhinny & Brock, 1975, from northwest and southern Africa, form a cluster whose distribution was considered to be typical of poles sampled from regions of continental extent. This led McElhinny & Brock, 1975, to conclude that the palaeopole position, with respect to Africa, remained in essentially the same position throughout the whole of the Mesozoic.

The method of minimal dispersion of VGPs set out by Ward (1963) was applied to the African Triassic and Cretaceous groups of data separately to determine the mean pole position, and a close approximation to the palaeoradius determined using the palaeomeridian method of Egyed (1960) where:
 

The data of McElhinny & Brock (1975) differ from the European data of Egyed (1960) and Cox & Doell (1961a) in that, the sample sites from Africa straddle the palaeoequator. As such, the latitudes calculated from site-data were added together rather than subtracted as in the European case.

The value of R_a determined by McElhinny & Brock (1975) amounted to 1.11 ± 0.20 for the Triassic, 1.06 ± 0.24 for the Cretacious, and 1.12 ± 0.17 for all of the Mesozoic values combined. These may be compared to values of 1.08 for the Triassic and 1.03 for the Cretacious determined by McElhinny & Brock (1975), using Fisher statistics to determine the palaeoradius from a pole precision (k) maxima plot. The values of R_a determined demonstrated to McElhinny & Brock (1975) that hypotheses of Earth expansion "are very difficult to sustain".

Figure 57 McElhinny & Brock's (1975) African Mesozoic site locations (lower figure) and palaeomagnetic pole positions (upper figure) used to determine palaeoradius of the Earth. Full circles represent Triassic, and full squares represent Cretaceous site locations and pole positions in both figures. (From McElhinny & Brock, 1975)

At first glance McElhinny & Brock's (1975) determination of palaeoradius appears conclusive. Africa is generally considered geologically stable therefore site separation may be deemed constant, pole positions form a relatively neat cluster and the R_a/R ratios determined from two independent methods consistently indicated nil expansion. Like Egyed (1960), Cox & Doell (1961a, 1961b), van Hilten (1963, 1968) and Ward (1963) the primary assumption in determining palaeoradius was the constancy of continental surface area. As previously demonstrated the conventional palaeomeridian method is insensitive to crustal extension, and the method of minimal dispersion assumes that the most probable palaeoradius is determined from the point of minimal dispersion of poles. In addition it must be noted that the fundamental dipole equation determines latitude/colatitude only from site mean inclination data. The dipole equation cannot determine longitude, hence any rotation of either or both of the site locations relative to each other cannot be detected using the palaeomeridian method.

Rotation of the site locations will however decrease the meridional angular separation, while still retaining a constant angular separation and constant pole position. Intracontinental deformation of Africa was documented independently by Unternehr et al (1988), which complements observations from empirical small Earth modeling (Figure 25) suggesting the possibility of intracratonic plate rotation for Africa.This rotation allowed for a better reconstruction for South America and Africa along the South Atlantic spreading axis in both conventional and small Earth reconstructions.

The results of McElhinny & Brock (1975) therefore cannot be considered conclusive of zero Earth expansion with time, and in fact can be equally shown to be evidence for expansion.

Back to Contents

Discussion

The geological implications of an expanding Earth, as can be appreciated, is a topic which requires as much geological input and research as has plate tectonics to do it justice. The subject is further discussed in Carey (1975, 1988, 1994) (see David Ford's web site "The EXPANDING EARTH"), covering a broad range of topics not included in this paper. The brief discussions on relief of surface curvature, orogenesis, atmospheric and hydrospheric accumulation, and palaeomagnetics were addressed as the major perceived problems confronting the general acceptance of Earth expansion as a viable alternative to plate tectonics.

During Earth expansion, when dealing with relief of surface curvature, it was considered important to recognize the broad tectonic distribution of cratons, orogens, and sedimentary basins, each with their own definitional tectonic framework, which differ fundamentally from the modern ocean basin setting. Small Earth modeling demonstrated that each of these tectonic regimes react differently during Earth expansion, from fragmentation of Precambrian cratons, such as the Canadian Arctic Islands and Greenland, to large scale continental extension marked by intracratonic sedimentary basins, such as the West Siberian Basin in central Russia.

Orogenesis was considered intimately related to asymmetric expansion of the Earth resulting in intracratonic interaction during relief of surface curvature. This asymmetric expansion process was attributted to the hemihedral and antipodal distribution of the present day continents and oceans. The radial and tangential vector components of this asymmetric expansion process giving rise to a continuum of orogenic models varying from compressional to translational and torsional.

Similarly, during Earth expansion, it was suggested that the whole column of atmosphere, hydrosphere, oceanic lithosphere and underlying mantle has been added at an accelerating rate through geological time, which Carey (1983c) considered to have been accreted primarily at the growing ridges and rift zones. As the ocean waters and ocean floors both have the same origin it is to be expected that they would be produced pari passu, with the generation of ocean water and atmosphere keeping pace with the growth of oceanic lithosphere (Carey, 1983c).

Palaeomagnetics has long been considered the cornerstone of plate tectonics yet fundamental premises regarding the constancy of continental surface area, used to determine palaeoradius, stem from the early 1960s, prior to the development of modern global tectonic concepts or completion of the oceanic crustal database. Mathematical equations were developed by palaeomagneticians from conclusions that insist that continental surface areas have remained essentially constant, hence any variation in palaeoradius was concluded to have been negligible with time. Since these equations were first derived, modern plate tectonic concepts have demonstrated that the Earth's crust is not a passive adjunct of lithospheric plates, but a dynamic, interactive layer of the Earth (Grant, 1992).

The modified palaeomagnetic equations developed in this web site for the determination of palaeopole positions since the Early Jurassic prompts a need for a more thorough overhaul of the concepts of palaeomagnetics, in particular the conclusions drawn from the interpretations of pole positions, apparent polar wander paths, and displaced terranes.

Back to Contents